Simulation Inputs

Simulation Inputs

Simulation

 

Predicting metal flow and failure is the essence of sheet metal forming simulation.  Characterizing the stress-strain response to metal flow requires a detailed understanding of when the sheet metal first starts to permanently deform (known as the yield criteria), how the metal strengthens with deformation (the hardening law), and the failure criteria (for example, the forming limit curve). Complicating matters is that each of these responses changes as three-dimensional metal flow occurs, and are functions of temperature and forming speed. 

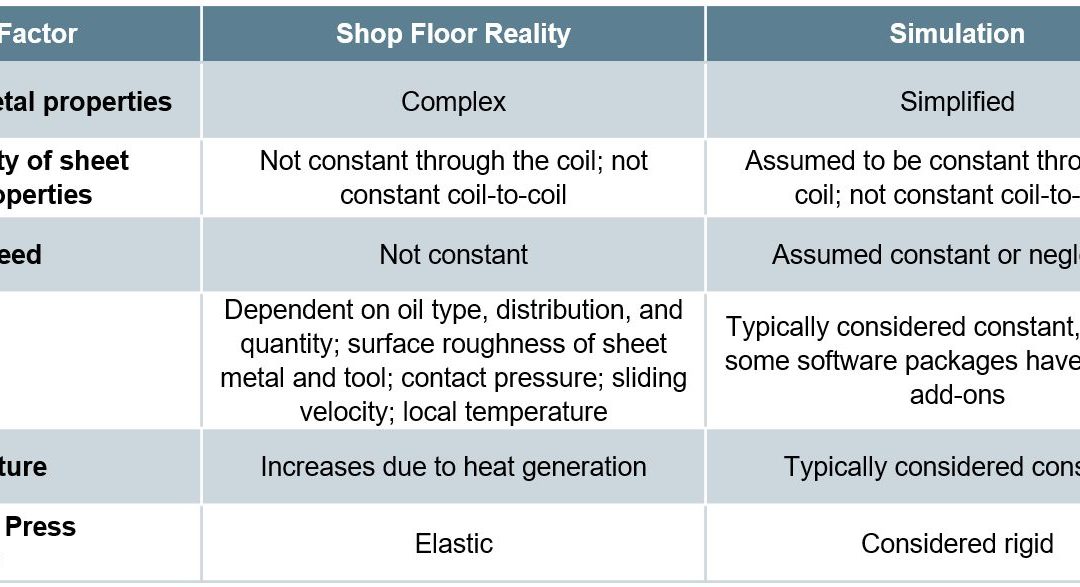
The ability to simulate these features reliably and accurately requires mathematical constitutive laws that are appropriate for the material and forming environments encountered. Advanced models typically improve prediction accuracy, at the cost of additional numerical computational time and the cost of experimental testing to determine the material constants. Minimizing these costs requires compromises, with some of these indicated in Table I created based on Citation R-28.

Table I: Deviations from reality made to reduce simulation costs. Based on Citations B-16 and R-28.

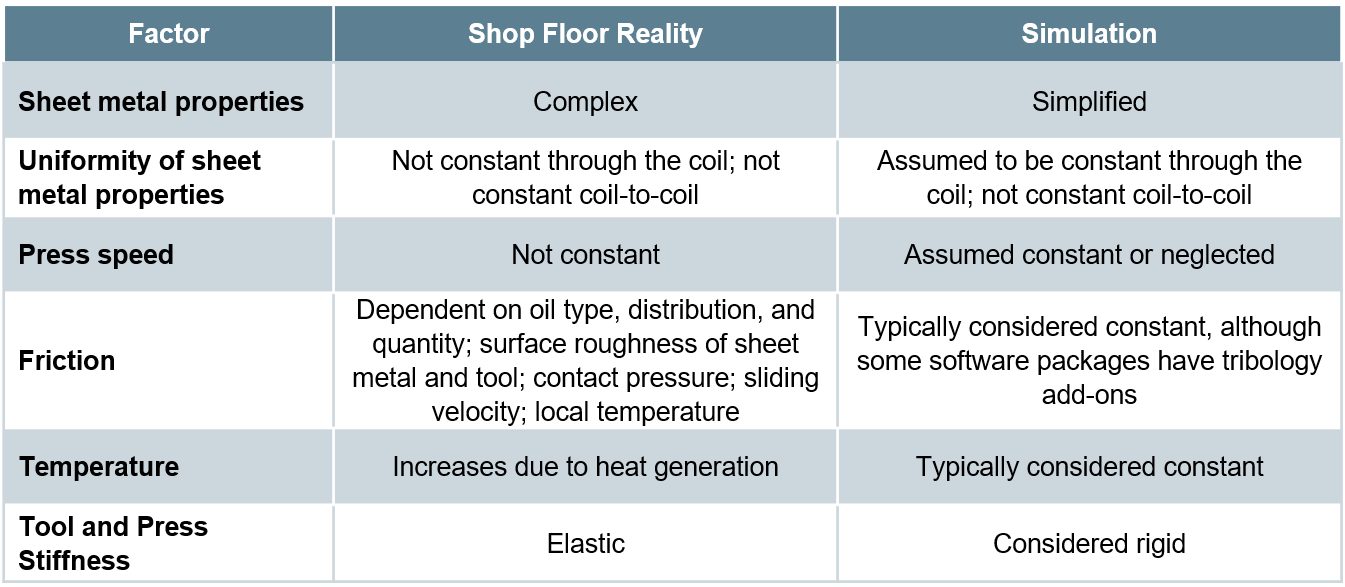
Table I: Deviations from reality made to reduce simulation costs. Based on Citation R-28.

 

Yield Criteria

The yield criteria (also known as the yield surface or yield loci) defines the conditions representing the transition from elastic to plastic deformation.  Assuming uniform metal properties in all directions allows for the use of isotropic yield functions like von Mises or Tresca. A more realistic approach considers anisotropic metal flow behavior, requiring the use of more complex yield functions like those associated with Hill, Barlat, Banabic, or Vegter.   

No one yield function is best suited to characterize all metals. Some yield functions have many required inputs.  For example, “Barlat 2004-18p” has 18 separate parameters leading to improved modeling accuracy – but only when inserting the correct values. Using generic textbook values is easier, but negates the value of the chosen model.  However, determining these variables typically is costly and time-consuming, and requires the use of specialized test equipment.

 

Hardening Curve

Metals get stronger as they deform, which leads to the term work hardening. The flow stress at any given amount of plastic strain combines the yield strength and the strengthening from work hardening.  In its simplest form, the stress-strain curve from a uniaxial tensile test shows the work hardening of the chosen sheet metal. This approach ignores many of the realities occurring during forming of engineered parts, including bi-directional deformation.

Among the simpler descriptions of flow stress are those from Hollomon, Swift, and Ludvik.  More complex hardening laws are associated with Voce and Hockett-Sherby. 

The strain path followed by the sheet metal influences the hardening. Approaches taken in the Yoshida-Uemori (YU) and the Homogeneous Anisotropic Hardening (HAH) models extend these hardening laws to account for Bauschinger Effect deformations (the bending-unbending associated with travel over beads, radii, and draw walls).

As with the yield criteria, accuracy improves when accounting for three-dimensional metal flow, temperature, and forming speed, and using experimentally determined input parameters for the metal in question rather than generic textbook values. 

 

Failure Conditions

Defining the failure conditions is the other significant challenge in metal forming simulation. Conventional Forming Limit Curves describe necking failure under certain forming modes, and are easier to understand and apply than alternatives. Complexity and accuracy increase when accounting for non-linear strain paths using stress-based Forming Limit Curves.  Necking failure is not the only type of failure mode encountered. Conventional FLCs cannot predict fracture on tight radii and cut edges, nor can they account for dimensional issues like springback.  For these, failure criteria definitions which are more mathematically complex are appropriate.

 

Constitutive Laws

Simple material models reduce the effort of testing, but may not be sufficiently accurate or do not apply to the spectrum of grades available today.

The yield surface constructed with Hill’48H-74 requires only data from three tensile tests. Models like Barlat-1989B-89, Barlet-2003B-90, Barlat-2005B-91,  BBC2005B-92, VegterV-26, and Vegter liteV-27 all require data from more detailed advanced tests, but simulation results incorporating these yield surfaces more closely match experimental results.

Use caution with the assumptions that go into the Material Card.  For example, the card may show the r-value in the rolling, diagonal, and transverse (0°, 45°, and 90°) orientations all the same (not realistic), or worse yet, all equal to 1. For high strength and advanced high strength steels, it is likely that at least one of the orientations will have an r-value below 1. In these cases assuming an r-value of 1 will lead to an underestimation of the thinning.  Furthermore, use of the Hill 1948 yield criterion is not recommended since the model assumptions do not apply to r-values of less than 1.

The Keeler equation for FLC0 K-71 requires only the n-value and thickness, but is based on a correlation established from grades and testing available no later than the early 1990s.

Predictive models for the yield surfaceA-96 and FLCsA-97 in cold stamping conditions were created to simplify the testing requirements while maintaining the accuracy and usefulness of these advanced models. These predictive equations have been validated against physical testing for mild steels, conventional high strength steels, advanced high strength steels, aluminum alloys, and stainless steel grades. 

Similarly, for hot stamping, predictive models based on conventional tensile testing have been developed and verified.A-94, D-48, A-98  Challenges here include that elongation and r-value both vary with temperature and testing speed.

On the Forming Limit Curve shown in Figure 1a, the uniaxial strain path, plane strain, biaxial, and balanced biaxial points are predicted from total elongation (A80) and r-value determined from tensile testing in the 0, 45 and 90° orientations with respect to rolling direction.A-94 Compared with the Keeler model, the Abspoel & Scholting model is better at predicting the FLC of DP800, noting the upper limiting strains found in the experiment match the FLC, as well as a more accurate representation of the slope on the LH side.  Both models appear sufficient for the conventional grade DC04 (similar to CR3).

Figure 1. a) FLC prediction locations. b) FLC comparison on drawing steel DC04 (CR3); c) FLC comparison on DP800.A-94

Figure 1. a) FLC prediction locations. b) FLC comparison on drawing steel DC04 (CR3); c) FLC comparison on DP800.A-94

 

Yield surface correlations use Tensile strength (Rm), uniform elongation (Ag) and r-value test data as inputs to predict the equi-biaxial, plane strain and shear points in three directions. Figure 2 compares biaxial yield strength predictions between Hill’48 and Vegter 2017, showing the improved correlation in the model developed almost 70 years later.

Figure 2. Comparison of measured biaxial yield strength with prediction from Hill’48 (red) and Vegter 2017 (yellow).A-94

Figure 2. Comparison of measured biaxial yield strength with prediction from Hill’48 (red) and Vegter 2017 (yellow).A-94

 

Figure 3 presents a comparison of the measured yield surfaces of DX54D+Z (galvanized CR3) and DP1000 with those predicted by Hill’48 and Vegter 2017, highlighting the improved accuracy found in Vegter 2017.

Figure 3. Comparison of measured yield surface with predictions from Hill’48 and Vegter 2017. a) DX54D+Z (galvanized CR3); b) DP1000.A-94

Figure 3. Comparison of measured yield surface with predictions from Hill’48 and Vegter 2017. a) DX54D+Z (galvanized CR3); b) DP1000.A-94

 

Material properties like elongation, r-value, the hardening curve, and forming limits are all likely both strain-rate and temperature dependent, meaning that a rate-dependent and temperature-dependent yield surface and forming limit curve are needed for more accurate representations of cold- and hot-stamping.

The initial implementation of the Vegter yield surface in forming simulation software packages showed satisfactory correlation with conventional stamping applications, but was sub-optimal in operations where stresses are found in the thickness direction such as coining, wall ironing and score forming processes in packaging and battery applications. In these cases, a non-convexity close to the equi-biaxial point of the yield locus was observed, likely due to extreme anisotropy or very low r-values.

Citation A-95 discusses methods for improved accuracy. DIC measurements offer improved r-value characterization over mechanical measurement approaches. Yield locus correlations at low and high plastic strain ratios were also improved. Shell elements used in the simulation of the yield surface in plane stress ignore the strains in the through-thickness direction. For the applications where thickness stresses play a role (like wall ironing, coining, score forming in packaging, and sharp radii in closures, solid or thick shell elements are required.  The yield surface was extended to the thickness direction to allow for improved characterization in these applications having significant stresses through the thickness. This extension may be deployed in forming simulation software as “Vegter 2017.1.”

 

Constitutive Laws and Their Influence on Forming Simulation Accuracy

Many simulation packages allow for an easy selection of constitutive laws, typically through a drop-down menu listing all the built-in choices. This ease potentially translates into applying inappropriate selections unless the simulation analyst has a fundamental understanding of the options, the inputs, and the data generation procedures.

Some examples:

  • The “Keeler Equation” for the estimation of FLC0 has many decades of evidence in being sufficiently accurate when applied to mild steels and conventional high strength steels. The simple inputs of n-value and thickness make this approach particularly attractive.  However, there is ample evidence that using this approach with most advanced high strength steels cannot yield a satisfactory representation of the Forming Limit Curve.
  • Even in cases where it is appropriate to use the Keeler Equation, a key input is the n-value or the strain hardening exponent. This value is calculated as the slope of the (natural logarithm of the true stress):(natural logarithm of the true strain curve). The strain range over which this calculation is made influences the generated n-value, which in turn impacts the calculated value for FLC0.
  • The strain history as measured by the strain path at each location greatly influences the Forming Limit. However, this concept has not gained widespread understanding and use by simulation analysts.
  • A common method to experimentally determine flow curves combines tensile testing results through uniform elongation with higher strain data obtained from biaxial bulge testing. Figure 4 shows a flow curve obtained in this manner for a bake hardenable steel with 220 MPa minimum yield strength.  Shown in Figure 5 is a comparison of the stress-strain response from multiple hardening laws associated with this data, all generated from the same fitting strain range between yield and tensile strength.  Data diverges after uniform elongation, leading to vastly different predictions. Note that the differences between models change depending on the metal grade and the input data, so it is not possible to say that one hardening law will always be more accurate than others.
Figure 1: Flow curves for a bake hardenable steel generated by combinng tensile testing with bulge testing L-20

Figure 4: Flow curves for a bake hardenable steel generated by combining tensile testing with bulge testing.L-20

 

Figure 2: The chosen hardening law leads to vastly different predictions of stress-strain responses L-20

Figure 5: The chosen hardening law leads to vastly different predictions of stress-strain responses.L-20

 

  • Analysts often treat Poisson’s Ratio and the Elastic Modulus as constants.  It is well known that the Bauschinger Effect leads to changes in the Elastic Modulus, and therefore impacts springback.  However, there are also significant effects in both Poisson’s Ratio (Figure 6) and the Elastic Modulus (Figure 7) as a function of orientation relative to the rolling direction. Complicating matters is that this effect changes based on the selected metal grade.  
Figure 3:  Poisson’s Ratio as a Function of Orientation for Several Grades (Drawing Steel, DP 590, DP 780, DP 1180, and MS 1700) D-11

Figure 6:  Poisson’s Ratio as a Function of Orientation for Several Grades (Drawing Steel, DP 590, DP 980, DP 1180, and MS 1700) D-11

 

Figure 4:  Modulus of Elasticity as a Function of Orientation for Several Grades (Drawing Steel, DP 590, DP 780, DP 1180, and MS 1700) D-11

Figure 7:  Modulus of Elasticity as a Function of Orientation for Several Grades (Drawing Steel, DP 590, DP 980, DP 1180, and MS 1700) D-11

 

Testing to Determine Inputs for Simulation

Complete material card development requires results from many tests, each attempting to replicate one or more aspects of metal flow and failure. Certain models require data from only some of these tests, and no one model typically is best for all metals and forming conditions.  Tests described below include:

  • Tensile testing [room temperature at slow strain rates to elevated temperature with accelerated strain rates]
  • Biaxial bulge testing
  • Biaxial tensile testing
  • Shear testing
  • V-bending testing
  • Tension-compression testing with cyclic loading
  • Friction

Tensile testing is the easiest and most widely available mechanical property evaluation required to generate useful data for metal forming simulation. However, a tensile test provides a complete characterization of material flow only when the engineered part looks like a dogbone and all deformation resulted from pulling the sample in tension from the ends. That is obviously not realistic. Getting tensile test results in more than just the rolling direction helps, but generating those still involves pulling the sample in tension.  Three-dimensional metal flow occurs, and the stress-strain response of the sheet metal changes accordingly.  

The uniaxial tensile test generates a draw deformation strain state since the edges are free to contract.  A plane strain tensile test requires using a modified sample geometry with an increased width and decreased gauge length, 

Forming all steels involves a thermal component, either resulting from friction and deformation during “room temperature” forming or the intentional addition of heat such as used in press hardening. In either case, modeling the response to temperature requires data from tests occurring at the temperature of interest, at appropriate forming speeds.  Thermo-mechanical simulators like Gleeble™ generate such data.

Conventional tensile testing occurs at deformation rates of 0.001/sec. Most production stamping occurs at 10,000x that amount, or 10/sec. Crash events can be 2 orders of magnitude faster, at about 1000/sec.  The stress-strain response varies by both testing speed and grade. Therefore, accurate simulation models require data from higher-speed tensile testing. Typically, generating high speed tensile data involves drop towers or Split Hopkinson Pressure Bars.

A pure uniaxial stress state exists in a tensile test only until reaching uniform elongation and the beginning of necking.  Extrapolating uniaxial tensile data beyond uniform elongation risks introducing inaccuracies in metal flow simulations. Biaxial bulge testing generates the data for yield curve extrapolation beyond uniform elongation. This stretch-forming process deforms the sheet sample into a dome shape using hydraulic pressure, typically exerted by water-based fluids.  Citation I-12 describes a standard test procedure for biaxial bulge testing.

A Marciniak test used to create Forming Limit Curves generates in-plane biaxial strains.  Whereas FLC generation uses 100 mm diameter samples, larger samples allow for extraction of full-size tensile bars.  Although this approach generates samples containing biaxial strains, the extracted samples are tested uniaxially in the conventional manner.

Biaxial tensile testing allows for the determination of the yield locus and the biaxial anisotropy coefficient, which describes the slope of the yield surface at the equi-biaxial stress state. This test uses cruciform-shaped test pieces with parallel slits cut into each arm. Citation I-13 describes a standard test procedure for biaxial tensile testing.  The biaxial anisotropy coefficient can also be determined using the disk compression testing as described in Citation T-21.

Shear testing characterizes the sheet metal in a shear loading condition. There is no consensus on the specimen type or testing method. However, the chosen testing set-up should avoid necking, buckling, and any influence of friction.

V-bending tests determine the strain to fracture under specific loading conditions. Achieving plane strain or plane stress loading requires use of a test sample with features promoting the targeted strain state. 

Tension-compression testing characterizes the Bauschinger Effect.  Multiple cycles of tension-compression loading captures cyclic hardening behavior and elastic modulus decay, both of which improve the accuracy of springback predictions. 

The Bauschinger effect leads to early re-yielding after loading reversal, and has been observed in loading-reverse loading testing.  The Yoshida-Uemori (YU) kinematic hardening model accurately captures the Bauschinger effect as well as other hardening behaviors of sheet metals during loading-reverse loading.Y-7, Y-8

Characteristics of the Bauschinger Effect (Figure 8) include a) the transient Bauschinger deformation characterized by early re-yielding and smooth elastic–plastic transition with a rapid change of the work hardening rate; b) the permanent softening characterized by a stress offset observed in a region after the transient period; and c) work-hardening stagnation appearing at a certain range of reverse deformation.Y-7

Figure 5: Characteristics of the Bauschinger Effect during cyclic loading. Y-7

Figure 8: Characteristics of the Bauschinger Effect during cyclic loading.Y-7

 

Citation L-78 describes an approach to calibrate the YU model on a QP1500 steel that uses a combination of physical testing and machine learning to achieve loading-reverse loading stress-strain curves over broader strain ranges.  This citation also reported that the results from tension-compression testing were not the same as those from compression-tension testing – meaning that the order of deformation influences the results.

However, no standard procedure exists for determining the kinematic hardening and Bauschinger parameters and subsequently incorporating it into metal forming simulation codes. Independent of the procedure, one of the biggest challenges with this test is preventing buckling from occurring during in-plane compressive loading. Related to this is the need to compensate for the friction caused by the anti-buckling mechanism in the stress-strain curves.

Friction is obviously a key factor in how metal flows.  However, there is no one simple value of friction that applies to all surfaces, lubricants, and tooling profiles. The coefficient of friction not only varies from point to point on each stamping but changes during the forming process. Determining the coefficient of friction experimentally is a function of the testing approach used. The method by which analysts incorporate friction into simulations influences the accuracy and applicability of the results of the generated model.

Studies are underway to reduce the costs and challenges of obtaining much of this data. It may be possible, for example, to use Digital Image Correlation (DIC) during a simple uniaxial tensile testing to quantify r-value at high strains, determine the material hardening behavior along with strain rate sensitivity, assess the degradation of Young’s Modulus during unloading, and use the detection of the onset of local neck to help account for non-linear strain path effects.S-110

 

Application of Advanced Testing to Failure Predictions

Global formability failures occur when the forming strains exceed the necking forming limit throughout the entire thickness of the sheet. Advanced steels are at risk of local formability failures where the forming strains exceed the fracture forming limit at any portion of the thickness of the sheet.

Fracture forming limit curves plot higher than the conventional necking forming limit curves on a graph showing major strain on the vertical axis and minor strain on the horizontal axis.  In conventional steels the gap between the fracture FLC and necking FLC is relatively large, so the part failure is almost always necking.  The forming strains are not high enough to reach the fracture FLC.

In contrast, AHSS grades are characterized by a smaller gap between the necking FLC and the fracture FLC.  Depending on the forming history, part geometry (tight radii), and blank processing (cut edge quality), forming strains may exceed the fracture FLC at an edge or bend before exceeding the necking FLC through-thickness.  In this scenario, the part will fracture without signs of localized necking.

A multi-year study funded by the American Iron and Steel Institute at the University of Waterloo Forming and Crash Lab describes a methodology used for forming and fracture characterization of advanced high strength steels, the details of which can be found in Citations B-11, W-20, B-12, B-13, R-5, N-13 and G-19.

This collection of studies, as well as work coming out of these studies, show that relatively few tests sufficiently characterize forming and fracture of AHSS grades.  These studies considered two 3rd Gen Steels, one with 980MPa tensile strength and one with 1180MPa tensile.

  • The yield surface as generated with the Barlat YLD2000-2d yield surface (Figure 9) comes from:
    • Conventional tensile testing at 0, 22.5, 45, 67.5, and 90 degrees to the rolling direction, determining the yield strength and the r-value;
    • Disc compression tests according to the procedure in Citation T-21 to determine the biaxial R-value, rb.
Figure 5: Tensile testing and disc compression testing generate the Barlat YLD2000-2d yield surface in two 3rd Generation AHSS Grades B-13

Figure 9: Tensile testing and disc compression testing generate the Barlat YLD2000-2d yield surface in two 3rd Generation AHSS Grades B-13

 

  • Creating the hardening curve uses a procedure detailed in Citations R-5 and N-13, and involves only conventional tensile and shear testing using the procedure included in Citation P-15.
Figure 6: Test geometries for hardening curve generation. Left image: Tensile; Right image: Shear.  N-13

Figure 10: Test geometries for hardening curve generation. Left image: Tensile; Right image: Shear.N-13

 

  • Characterizing formability involved generating a Forming Limit Curve using Marciniak data or process-corrected Nakazima data. (See our article on non-linear strain paths) and Citation N-13 for explanation of process corrections].  Either approach resulted in acceptable characterizations.
  • Fracture characterization uses four plane stress tests: shear, conical hole expansion, V-bending, and a biaxial dome test.  The result from these tests calibrate the fracture locus describing the stress states at fracture.

 

A different approach requires only the results from conventional tensile testing and a crack growth test under simple loading to simulate post-necking strain hardening behavior and ductile fracture.  The details of this approach are beyond the scope of this webpage, but are presented in detail in Citations S-124 and T-56, in addition to verification procedures.

 

Simulation Set-Up Parameters

One of the most basic choices when starting a simulation run is the setting related to the mesh size. Reduced processing time is associated with large mesh sizes, but that risks not having sufficiently fine mesh resolution to capture the forming strain gradient.  A large mesh size averages the strains over a larger region, which is analogous to a tensile bar with an 80 mm gauge length having lower elongation than a 50 mm tensile bar cut from the same sheet steel.

Figure 11 compares the Forming Limit Curve (FLC) for an 1180 MPa steel determined from gauge lengths of 2, 6, and 10 mm, along with the associated theoretical predictions. As expected, the smaller gauge length is able to more effectively capture peak strains, and is therefore associated with a higher forming limit.A-90

Figure 7: Comparison of predicted values and experimental values of the Forming Limit Curve of an 1180 MPa steel. A-90

Figure 11: Comparison of predicted values and experimental values of the Forming Limit Curve of an 1180 MPa steel.A-90

 

The stress-strain curve of a 1.6 mm 980 MPa steel tested with a 50 mm gauge length (ISO III, JIS) was captured, resulting in a strain at fracture of 0.147. A model based on a 2 mm element size was created, calibrated to the same strain at fracture of 0.147.  The model was re-run with element sizes of 3 mm and 5 mm, which resulted in different stress strain curves and simulations that could not predict the fracture known to occur, Figure 12. This study also showed a technique that can be used to achieve similar performance nearly independent of mesh size, such that accuracy is not compromised when optimizing computer processing speed. A-90

Figure 8: Comparison of the tensile test result and fracture model predictions based on different element sizes. A-90

Figure 12: Comparison of the tensile test result and fracture model predictions based on different element sizes.A-90

 

Constitutive Models

Constitutive models for steel strengthening fall into two general categories:  power law behavior like HollomonH-71 and SwiftS-119 or saturation models like Voce and Hockett-Sherby.H-72  As shown in Figure 2 above, the chosen constitutive model significantly influences the extrapolation of experimental stress-strain curves to larger strain values. Model combinations such as Swift-Voce or Swift/Hockett-Sherby, typically using one for lower strains and the other for higher strains, typically provide better fit with experimental dataK-65, but more parameters are usually beneficial, especially for advanced high strength steels where the n-value is not constant with strain.

To improve the modeling accuracy of high strength steels with variable instantaneous n–value, hardening curves obtained with uniaxial tensile and hydraulic bulge tests were fit to a new proposed modelL-73 to verify its predictive capability and accuracy. This new model, based on the Swift power law (Equation 1), addresses the decrease in n-value at larger plastic strains by varying what has been termed as the strain hardening attenuation coefficient a, within a new parameter λh as defined in Equation 2.

Equation 1
Equation 1
Equation 2 in Sim
Equation 2

 
When a=0, Equation 1 reverts to the standard Swift equation. When a>0, it allows for Equation 1 to correct for the decrease of instantaneous n value occurring at larger plastic strains.  The results in Figure 133 show that the predictive accuracy of the new model is better than the individual Swift or Hockett-Sherby models.

Figure 7: Hardening Curve for two grades showing Uniaxial Tensile (ut) stress-strain curves, biaxial tensile extension from biaxial bulge testing (bt), Swift and Hockett-Sherby model fit, and new model fit with different a parameter. L-73

Figure 13: Hardening Curve for two grades showing Uniaxial Tensile (ut) stress-strain curves, biaxial tensile extension from biaxial bulge testing (bt), Swift and Hockett-Sherby model fit, and new model fit with different a parameter.L-73

 

Experimental hardening data for a QP grade, referred to as QP1180-EL, was obtained from uniaxial tensile testing combined with bulge testing and in-plane torsion testing for strains beyond uniform elongation.  These are shown as squares and black or red circles in Figure 14, along with projections from the Swift and Hockett-Sherby models.  The Modified Power Law (MPL) achieves the best fit to the tested results.Z-18

Figure 8: Experimental results compared with the Swift power law hardening model, Hockett-Sherby saturation hardening model, and a newly developed Modified Power Law.Z-18

Figure 14: Experimental results compared with the Swift power law hardening model, Hockett-Sherby saturation hardening model, and a newly developed Modified Power Law.Z-18

 

Improved vehicle crashworthiness predictions occur when the forming history of the critical structural parts, including the effects of bake hardening, work hardening, and thickness reduction, are incorporated into vehicle virtual development models. Historically, simulations did not contemplate the initial damage caused by plastic deformation.  Accumulated damage can be captured within a GISSMO (Generalized Incremental Stress State Dependent Model) damage model, albeit with certain assumptions.N-30, N-31

Five different loading cases capturing the stress state of shear, uniaxial tension, stretching, plane strain and equi-biaxial stretching can be used to calibrate parameters of the Modified Mohr-Coulomb (MMC) B-83 fracture model. Schematics of these five individual tests are shown in Figure 15.H-73   The calibrated MMC model and loading path results from these tests are shown in Figure 16.  The MMC model was subsequently used to calibrate a GISSMO damage model.

Figure 9: Tests coupons for fracture model calibration. H-73

Figure 15: Tests coupons for fracture model calibration.H-73

 

Figure 10. Calibrated MMC fracture model and loading path results from the tests shown in Figure 9. H-73

Figure 16. Calibrated MMC fracture model and loading path results from the tests shown in Figure 10.H-73

 

Quasi-static three-point quasi-static bending tests were used to validate the MPL hardening model, the MMC fracture model, and the GISSMO damage model.   An FEA model with a 2 mm mesh size was compared with one having a 5 mm mesh size for the simulation of the bending process. Figure 17 shows the predicted fracture location and test result.

Local necking was observed during the experimental bending test and 2 elements failed in when using a 2 mm mesh size, yet no failure was observed when using a 5 mm mesh. This indicates that accurate simulation results may require refined mesh sizes.

Figure 11: Experiment and simulation results of three-point bending testing of a Quenched & Partitioned 1180 MPa Steel H-73

Figure 17: Experiment and simulation results of three-point bending testing of a Quenched & Partitioned 1180 MPa Steel.H-73

 

A subsequent studyZ-18 confirmed that ignoring the stamping forming history in the damage model results in a lower prediction of the failure risk, especially for cold stamping high-strength steel parts under large deformation conditions.
 

Case Studies: Benefits of using Advanced Models for Springback Prediction

The output of simulations using material models that thoroughly capture the changes in metal properties occurring during forming are more likely to match reality than those simulations based on basic models.

Kinematic hardening models where the Bauschinger effect and modulus degradation are captured have been shown to be substantially more accurate in springback prediction than isotropic hardening models based on more conventional tensile testing.

Citation S-130 investigated this difference.  Different types of steels having 980 MPa or 1180 MPa minimum specified tensile strength from multiple suppliers were used to form a targeted part shape using either a draw forming process or a crash forming process. The formed panels were scanned and compared with simulation results from multiple software packages. In all cases, the simulation was capable of accurately predicting strains and the risk of necking failure. 

For springback, the type of hardening model used in the simulation appeared to correlate with prediction ability. Table 2 compares the dimensional difference between the model and a scan of the physical panel, with smaller numbers representing an improved ability to predict springback in the evaluated condition. As indicated in Table 2, models incorporating Kinematic Hardening more closely matched the actual springback seen on the scanned panels. 

Table 2: Dimensional Deviation Between Simulation and Scan as a Function of Hardening Model.S-130

Maximum Sectional Deviation
During Draw Forming (mm)		 Maximum Sectional Deviation
During Crash Forming (mm)		 Hardening Model
5.38 5.54 Isotropic Hardening
5.25 2.27 Yoshida
5.01 10.2 Isotropic Hardening
4.2 2.422 Hill ’48 Isotropic Hardening
4.17 3.127 Hill ’48 Isotropic Hardening
3.14 2.8 Yoshida
3.12 5.3 Yoshida
2.65 N/A Yoshida
2.3 4.2 Yoshida-Uemori
2.17 7.39 Yoshida
1.98 1.64 Yoshida-Uemori
1.797 1.952 Yoshida
1.5 2.3 Yoshida
Results compiled across multiple types of simulation software,
compared with formed parts made from different types of 980 and 1180 grades from multiple suppliers.

 

A modified S-shape generic panel was used to evaluate springback using a 3rd Generation Steel having a minimum specified tensile strength of 980MPa.B-98  Nearly 200 points were evaluated on the panel shown in Figure 18.  For the simulation that did not incorporate kinematic hardening, 48% of this panel were within 1 mm of the physical scanned part and 32% were within 0.5 mm.  When the simulation incorporated kinematic hardening, 94% of the points were within 1 mm and 60% were within 0.5 mm.

Figure 18: Modified S-Shape used to evaluate springback on 3rd Gen 980 MPa steel in Citation B-98.

Figure 18: Modified S-Shape used to evaluate springback on 3rd Gen 980 MPa steel in Citation B-98.

 

A generic B-pillar panel was used to evaluate springback using a 3rd Generation Steel having a minimum specified tensile strength of 1180MPa.K-72  

For the simulation that did not incorporate kinematic hardening, 59.9% of this panel were within 1 mm of the physical scanned part.  When the simulation incorporated kinematic hardening, 68.9% of the points were within 1 mm.  Simulation results are presented in Figure 19.

Figure 19: Springback results on a B-pillar formed from 3rd Gen 1180 MPa steel.K-72 https://ahssinsights.org/citations/k-72/

Figure 19: Springback results on a B-pillar formed from 3rd Gen 1180 MPa steel.K-72 

 

Back To Top

 

 

Dual Phase

Dual Phase

1stGen AHSS, AHSS, Steel Grades

Dual Phase (DP) steels have a microstructure consisting of a ferritic matrix with martensitic islands as a hard second phase, shown schematically in Figure 1. The soft ferrite phase is generally continuous, giving these steels excellent ductility. When these steels deform, strain is concentrated in the lower-strength ferrite phase surrounding the islands of martensite, creating the unique high initial work-hardening rate (n-value) exhibited by these steels. Figure 2 is a micrograph showing the ferrite and martensite constituents.

Figure 1: Schematic of a dual phase steel microstructure showing islands of martensite in a matrix of ferrite.

Figure 1: Schematic of a Dual Phase steel microstructure showing islands of martensite in a matrix of ferrite.

Figure 2: Micrograph of Dual Phase steel

Figure 2: Micrograph of Dual Phase Steel

Hot rolled DP steels do not have the benefit of an annealing cycle, so the dual phase microstructure must be achieved by controlled cooling from the austenite phase after exiting the hot strip mill finishing stands and before coiling. This typically requires a more highly alloyed chemistry than cold rolled DP steels require. Higher alloying is generally associated with a change in welding practices.

In one possible approach, after exiting the last finishing stand of the hot rolling mill, controlled cooling facilitates the nucleation of ferrite.  Then a more rapid cooling fast enough to avoid bainite formation is needed to reach the Ms (martensite start) temperature and begin nucleating martensite from the austenite that had not transformed to ferrite. 

Continuously annealed cold-rolled and hot-dip coated Dual Phase steels are produced by controlled cooling from the two-phase ferrite plus austenite (α + γ) region to transform some austenite to ferrite before a rapid cooling transforms the remaining austenite to martensite. Due to the production process, small amounts of other phases (bainite and retained austenite) may be present.

Higher strength dual phase steels are typically achieved by increasing the martensite volume fraction. Depending on the composition and process route, steels requiring enhanced capability to resist cracking on a stretched edge (as typically measured by hole expansion capacity) can have a microstructure containing significant quantities of bainite.

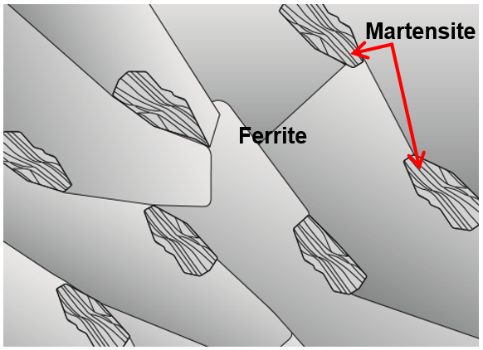
The work hardening rate plus excellent elongation creates DP steels with much higher ultimate tensile strengths than conventional steels of similar yield strength. Figure 3 compares the engineering stress-strain curve for HSLA steel to a DP steel curve of similar yield strength. The DP steel exhibits higher initial work hardening rate, higher ultimate tensile strength, and lower YS/TS ratio than the HSLA with comparable yield strength. Additional engineering and true stress-strain curves for DP steel grades are presented in Figures 4 and 5.

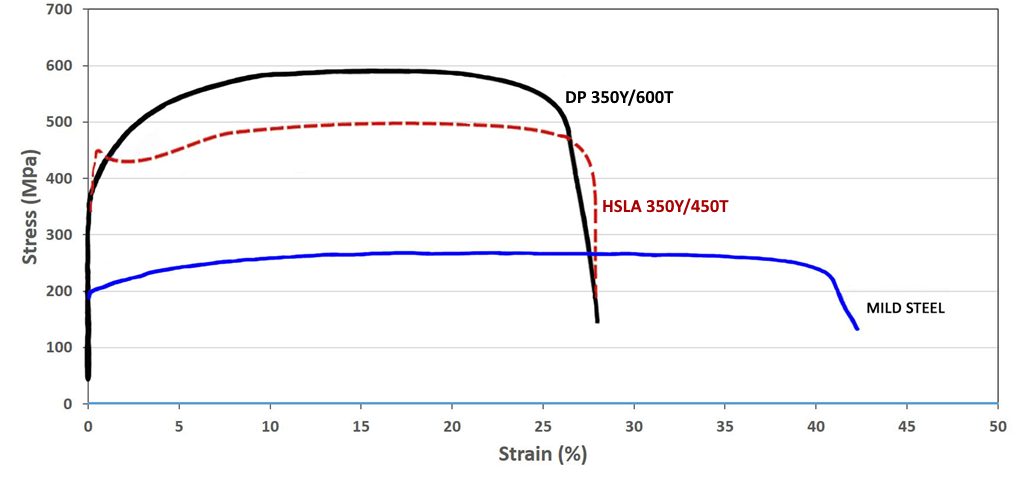
Figure 3: A comparison of stress strain curves for mild steel, HSLA 350/450, and DP 350/600

Figure 3: A comparison of stress strain curves for mild steel, HSLA 350/450, and DP 350/600K-1

 

Figure 4:  Engineering stress-strain curves for a series of DP steel grades.S-5, V-1  Sheet thicknesses: DP 250/450 and DP 500/800 = 1.0mm. All other steels were 1.8-2.0mm.

Figure 4:  Engineering stress-strain curves for a series of DP steel grades.S-5, V-1  Sheet thicknesses: DP 250/450 and DP 500/800 = 1.0mm. All other steels were 1.8-2.0mm.

 

Figure 5:  True stress-strain curves for a series of DP steel grades.S-5, V-1  Sheet thicknesses: DP 250/450 and DP 500/800 = 1.0mm. All other steels were 1.8-2.0mm.

Figure 5:  True stress-strain curves for a series of DP steel grades.S-5, V-1 Sheet thicknesses: DP 250/450 and DP 500/800 = 1.0mm. All other steels were 1.8-2.0mm.

 

The volume fraction, morphology, and distribution of the martensite in the ferrite matrix is responsible for the mechanical properties of dual phase (DP) steels. The intercritical annealing temperature, cooling rate, and alloy content affect the martensite volume fraction in the finished product.

Martensite can have different appearances (morphologies) in the microstructure including needle-like, granular, and equiaxed, and these impact the strength and ductility of DP steels.  The most favorable balance of strength and ductility usually is associated with a uniform distribution of equiaxed martensite islands.

These properties influence the hole expansion ratio, which measures the expandability of a sheared edge. The amount of carbon in martensite controls martensite hardness relative to the ferrite, and a greater hardness difference between martensite and ferrite is associated with decreased HER values.

The number of martensite colonies per unit area has a positive correlation with sheared edge stretchability, indicating that there is a greater dispersion of these islands of this high-hardness phase. A more homogeneous microstructure is known to have better HER and sheared-edge formability properties.T-57.    

Although dual phase steels are more formable than HSLA steels at the same tensile strength, there is a greater risk of cut edge fractures forming and propagating during stretch flanging. This is due to the hardness difference between the ferrite and martensite phases.

In these steels, micro-voids form at the interface between the soft phase and hard phase at the sheared edge (Figure 6), and can fracture during flanging under tension.  Reducing the hardness difference of the microstructural components is one approach to improve edge fracture resistance, which is one of the merits of using complex phase steels.

Figure 6: Microstructure at the punched edge of a DP steel.M-75

Figure 6: Microstructure at the punched edge of a DP steel.M-75

 

DP and other AHSS also have a bake hardening effect that is an important benefit compared to conventional higher strength steels. The extent of the bake hardening effect in AHSS depends on an adequate amount of forming strain for the specific chemistry and thermal history of the steel.

In DP steels, carbon enables the formation of martensite at practical cooling rates by increasing the hardenability of the steel. Manganese, chromium, molybdenum, vanadium, and nickel, added individually or in combination, also help increase hardenability. Carbon also strengthens the martensite as a ferrite solute strengthener, as do silicon and phosphorus. These additions are carefully balanced, not only to produce unique mechanical properties, but also to maintain the generally good resistance spot welding capability. However, when welding the higher strength grades (DP 700/1000 and above) to themselves, the spot weldability may require adjustments to the welding practice.

 

Dual Phase Steel for Exposed Panels

In recent decades, bake hardenable steels have been a common choice for outer surface panels.  Many of these applications center around grades with yield strength of approximately 200 MPa and tensile strength below approximately 400 MPa.  Work hardening (strengthening occurring from forming) combined with bake hardening (strengthening from the paint curing cycle during automotive production) usually adds around 70 to 100 MPa to the yield strength, enhancing the dent resistance of these panels.

To further support the lightweighting efforts of the automobile industry, steelmakers have developed dual phase steels with appropriate surface characteristics for exposed panel applications. The benefits of deploying dual phase steels in these applications include a higher yield strength from the steel mill (300 MPa minimum yield strength) and a greater strengthening increase from bake hardening (typically more than 100 MPa) in addition to the work hardening from forming.  The strengthening increase allows the automaker to downgauge the sheet thickness to as low as 0.55 mm and maintain adequate dent resistance.  More information on the bake hardenability of exposed quality dual phase steels can be found here.

The primary grade in this category can be described as HC300/500DPD+Z, where HC indicates that it is high strength cold rolled steel, 300/500 represents the minimum yield and tensile strength in MPa, DPD is “dual phase deep drawing,” and Z indicates that it is galvanized.

The stress-strain curves of HC300/500DPD+Z are compared with those of common traditional bake hardening grades used for automotive outer skin panels in Figures 7 and 8.  The comparison of engineering stress-strain curves are shown in Figure 7, with Figure 8 comparing the true stress-strain curves.

The dual phase steel exhibits a higher tensile strength and greater work hardening (n-value) – especially in the 4% to 6% range that coincides with the strain range associated with stamping automotive outer panels.

Figure 7: Engineering stress-strain curves for 0.6 mm HC300Y/500T-DPD+Z (galvanized 500 DP in red), 0.75 mm HC220BD+Z (galvanized 220 BH in blue), and 0.65 mm HC180BD+Z (galvanized 180 BH in black).

Figure 7: Engineering stress-strain curves for 0.6 mm HC300Y/500T-DPD+Z (galvanized 500 DP in red), 0.75 mm HC220BD+Z (galvanized 220 BH in blue), and 0.65 mm HC180BD+Z (galvanized 180 BH in black).

 

Figure 8: True stress-strain curves for 0.6 mm HC300Y/500T-DPD+Z (galvanized 500 DP in red), 0.75 mm HC220BD+Z (galvanized 220 BH in blue), and 0.65 mm HC180BD+Z (galvanized 180 BH in black).

Figure 8: True stress-strain curves for 0.6 mm HC300Y/500T-DPD+Z (galvanized 500 DP in red), 0.75 mm HC220BD+Z (galvanized 220 BH in blue), and 0.65 mm HC180BD+Z (galvanized 180 BH in black).

 

Figure 9 compares the forming limit curve for HC300/500DPD+Z steel to those of the typical bake hardenable grades. The dual phase grade has comparable to slightly less necking resistance than HC220BD+Z, a bake hardenable steel with 220 MPa minimum tensile strength.  The necking resistance of HC180BD+Z is greater than both other grades.

Figure 9: Forming limit curves for 0.6 mm HC300Y/500T-DPD+Z (galvanized 500 DP in red), 0.75 mm HC220BD+Z (galvanized 220 BH in blue), and 0.65 mm HC180BD+Z (galvanized 180 BH in black).

Figure 9: Forming limit curves for 0.6 mm HC300Y/500T-DPD+Z (galvanized 500 DP in red), 0.75 mm HC220BD+Z (galvanized 220 BH in blue), and 0.65 mm HC180BD+Z (galvanized 180 BH in black).

 

While the thickness reduction offered by HC300/500DPD+Z benefits lightweighting, there is also an associated loss of stiffness.  This reduced stiffness typically limits how thin automakers will specify for surface panels, rather than steel mill capabilities.

However, the lower stiffness, higher yield strength, and lower formability negatively influence dimensional accuracy and may contribute to welding challenges. Many of these challenges can be addressed virtually using metal forming simulation.

 

 

Examples of current production grades of DP steels and typical automotive applications include:

DP 300/500 Roof outer, door outer, body side outer, package tray, floor panel
DP 350/600 Floor panel, hood outer, body side outer, cowl, fender, floor reinforcements
DP 500/800 Body side inner, quarter panel inner, rear rails, rear shock reinforcements
DP 600/980 Safety cage components (B-pillar, floor panel tunnel, engine cradle, front sub-frame package tray, shotgun, seat)
DP 700/1000 Roof rails
DP 800/1180 B-Pillar upper

 

Some of the specifications describing uncoated cold rolled 1st Generation dual phase (DP) steel are included below, with the grades typically listed in order of increasing minimum tensile strength and ductility. Different specifications may exist which describe hot or cold rolled, uncoated or coated, or steels of different strengths. Many automakers have proprietary specifications which encompass their requirements.

  • ASTM A1088, with the terms Dual phase (DP) steel Grades 440T/250Y, 490T/290Y, 590T/340Y, 780T/420Y, and 980T/550YA-22
  • EN 10338, with the terms HCT450X, HCT490X, HCT590X, HCT780X, HCT980X, HCT980XG, and HCT1180XD-6
  • JIS G3135, with the terms SPFC490Y, SPFC540Y, SPFC590Y, SPFC780Y and SPFC980YJ-3
  • JFS A2001, with the terms JSC590Y, JSC780Y, JSC980Y, JSC980YL, JSC980YH, JSC1180Y, JSC1180YL, and JSC1180YHJ-23
  • VDA 239-100, with the terms CR290Y490T-DP, CR330Y590T-DP, CR440Y780T-DP, CR590Y980T-DP, and CR700Y980T-DPV-3
  • SAE J2745, with terms Dual Phase (DP) 440T/250Y, 490T/290Y, 590T/340Y, 6907/550Y, 780T/420Y, and 980T/550YS-18
Transformation Induced Plasticity (TRIP)

Transformation Induced Plasticity (TRIP)

1stGen AHSS, 3rdGen AHSS, AHSS, Steel Grades

topofpage

Metallurgy

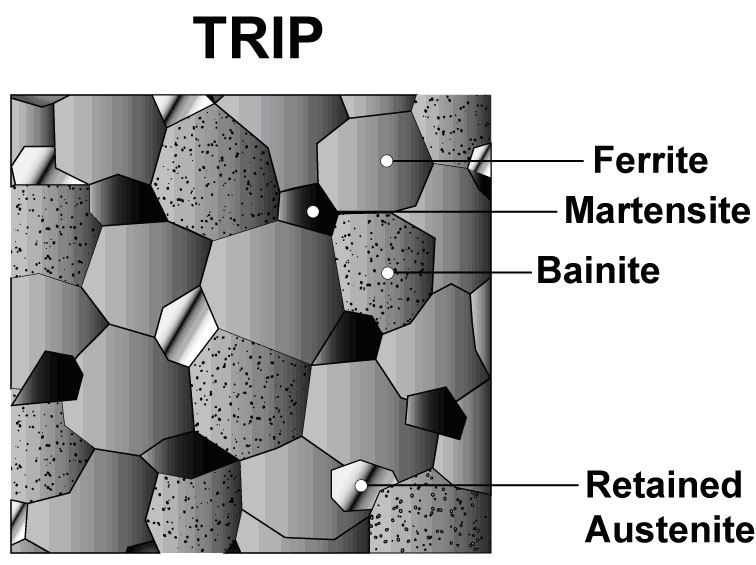
The microstructure of Transformation Induced Plasticity (TRIP) steels contains a matrix of ferrite, with retained austenite, martensite, and bainite present in varying amounts. Production of TRIP steels typically requires the use of an isothermal hold at an intermediate temperature, which produces some bainite. Higher silicon and carbon content of TRIP steels result in significant volume fractions of retained austenite in the final microstructure. Figure 1 shows a schematic of TRIP steel microstructure, with Figure 2 showing a micrograph of an actual sample of TRIP steel. Figure 3 compares the engineering stress-strain curve for HSLA steel to a TRIP steel curve of similar yield strength.

 

Figure 1: Schematic of a TRIP steel microstructure showing a matrix of ferrite, with martensite, bainite and retained austenite as the additional phases.

Figure 1: Schematic of a TRIP steel microstructure showing a matrix of ferrite, with martensite, bainite and retained austenite as the additional phases.

 

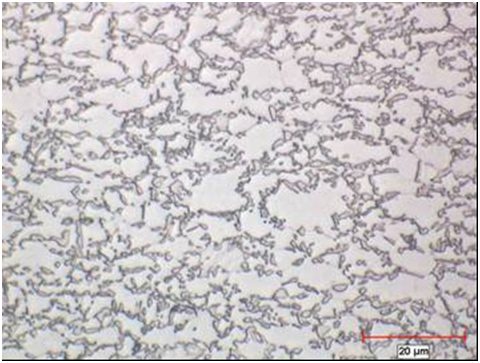
Figure 2: Micrograph of Transformation Induced Plasticity steel.

Figure 2: Micrograph of Transformation Induced Plasticity steel.

 

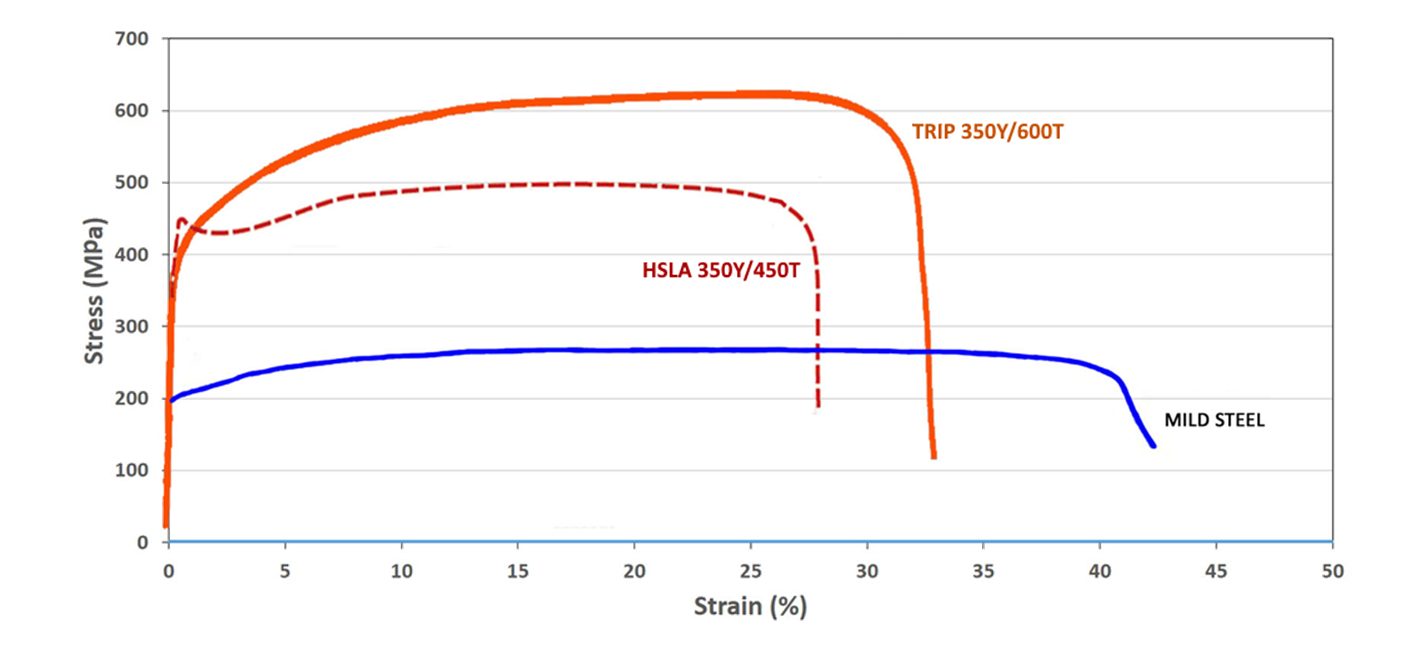
Figure 3: A comparison of stress strain curves for mild steel, HSLA 350/450, and TRIP 350/600.K-1

Figure 3: A comparison of stress strain curves for mild steel, HSLA 350/450, and TRIP 350/600.K-1

 

 

During deformation, the dispersion of hard second phases in soft ferrite creates a high work hardening rate, as observed in the DP steels. However, in TRIP steels the retained austenite also progressively transforms to martensite with increasing strain, thereby increasing the work hardening rate at higher strain levels. This is known as the TRIP Effect. This is illustrated in Figure 4, which compares the engineering stress-strain behavior of HSLA, DP and TRIP steels of nominally the same yield strength. The TRIP steel has a lower initial work hardening rate than the DP steel, but the hardening rate persists at higher strains where work hardening of the DP begins to diminish. Additional engineering and true stress-strain curves for TRIP steel grades are shown in Figure 5.

 

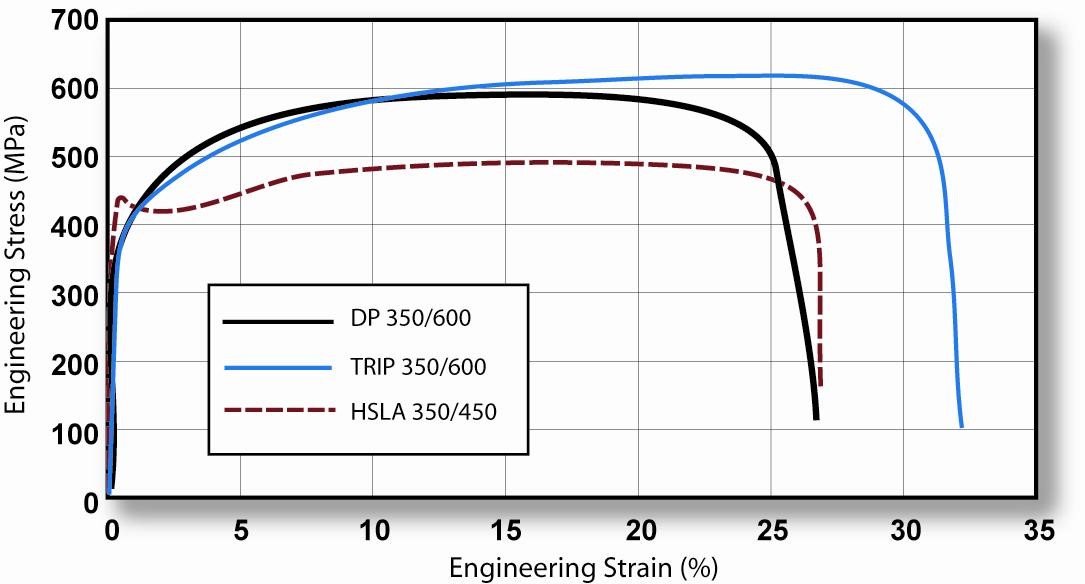
Figure 4: TRIP 350/600 with a greater total elongation than DP 350/600 and HSLA 350/450 Reference K-1

Figure 4: TRIP 350/600 with a greater total elongation than DP 350/600 and HSLA 350/450. K-1

 

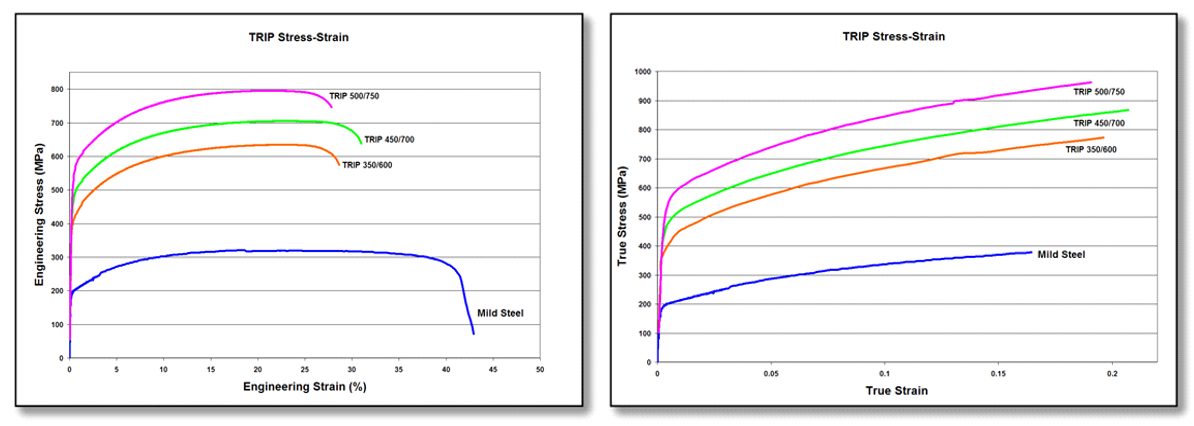
Figure 5: Engineering stress-strain (left graphic) and true stress-strain (right graphic) curves for a series of TRIP steel grades. Sheet thickness: TRIP 350/600 = 1.2mm, TRIP 450/700 = 1.5mm, TRIP 500/750 = 2.0mm, and Mild Steel = approx. 1.9mm. V-1

Figure 5: Engineering stress-strain (left graphic) and true stress-strain (right graphic) curves for a series of TRIP steel grades. Sheet thickness: TRIP 350/600 = 1.2mm, TRIP 450/700 = 1.5mm, TRIP 500/750 = 2.0mm, and Mild Steel = approx. 1.9mm. V-1

 

 

The strain hardening response of TRIP steels are substantially higher than for conventional HSS, resulting in significantly improved formability in stretch deformation. This response is indicated by a comparison of the n-value for the grades. The improvement in stretch formability is particularly useful when designers take advantage of the improved strain hardening response to design a part utilizing the as-formed mechanical properties. High n-value persists to higher strains in TRIP steels, providing a slight advantage over DP in the most severe stretch forming applications.

Austenite is a higher temperature phase and is not stable at room temperature under equilibrium conditions. Along with a specific thermal cycle, carbon content greater than that used in DP steels are needed in TRIP steels to promote room-temperature stabilization of austenite. Retained austenite is the term given to the austenitic phase that is stable at room temperature.

Higher contents of silicon and/or aluminum accelerate the ferrite/bainite formation. These elements assist in maintaining the necessary carbon content within the retained austenite. Suppressing the carbide precipitation during bainitic transformation appears to be crucial for TRIP steels. Silicon and aluminum are used to avoid carbide precipitation in the bainite region.

The carbon level of the TRIP alloy alters the strain level at which the TRIP Effect  occurs. The strain level at which retained austenite begins to transform to martensite is controlled by adjusting the carbon content. At lower carbon levels, retained austenite begins to transform almost immediately upon deformation, increasing the work hardening rate and formability during the stamping process. At higher carbon contents, retained austenite is more stable and begins to transform only at strain levels beyond those produced during forming. At these carbon levels, retained austenite transforms to martensite during subsequent deformation, such as a crash event.

TRIP steels therefore can be engineered to provide excellent formability for manufacturing complex AHSS parts or to exhibit high strain hardening during crash deformation resulting in excellent crash energy absorption.

The additional alloying requirements of TRIP steels degrade their resistance spot-welding behavior. This can be addressed through weld cycle modification, such as the use of pulsating welding or dilution welding.  Table 1 provides a list of current production grades of TRIP steels and example automotive applications:

Table 1: Current Production Grades Of TRIP Steels And Example Automotive Applications.

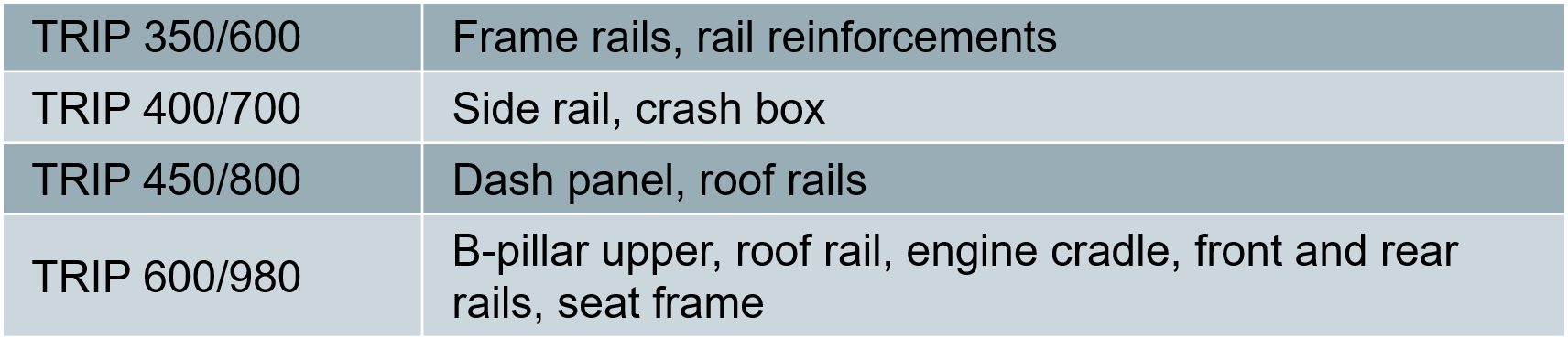
Table 1: Current Production Grades Of TRIP Steels And Example Automotive Applications.

 

Some of the specifications describing uncoated cold rolled 1st Generation transformation induced plasticity (TRIP) steel are included below, with the grades typically listed in order of increasing minimum tensile strength and ductility. Different specifications may exist which describe hot or cold rolled, uncoated or coated, or steels of different strengths. Many automakers have proprietary specifications which encompass their requirements.
• ASTM A1088, with the terms Transformation induced plasticity (TRIP) steel Grades 690T/410Y and 780T/440YA-22
• JFS A2001, with the terms JSC590T and JSC780TJ-23
• EN 10338, with the terms HCT690T and HCT780TD-18
• VDA 239-100, with the terms CR400Y690T-TR and CR450Y780T-TRV-3
• SAE J2745, with terms Transformation Induced Plasticity (TRIP) 590T/380Y, 690T/400Y, and 780T/420YS-18

 

 

Transformation Induced Plasticity Effect

Austenite is not stable at room temperature under equilibrium conditions. An austenitic microstructure is retained at room temperature with the combined use of a specific chemistry and controlled thermal cycle.

Deformation from sheet forming or from crash impact provides the necessary energy to allow the crystallographic structure to change from austenite to martensite. There is insufficient time and temperature for substantial diffusion of carbon to occur from carbon-rich austenite, which results in a high-carbon (high strength) martensite after transformation.  Strengthening also occurs from the dislocations formed in the adjacent ferrite required to accommodate the volume increase associated with the austenite-to-martensite transformation.

Transformation to high strength martensite continues as deformation increases, as long as retained austenite (RA) is still available to be transformed. Optimal combinations of strength and ductility are obtained when the retained austenite stability is such that the transformation to martensite occurs gradually with increasing strain.

Alloys capable of the TRIP effect are characterized by a high ductility – high strength combination. Such alloys include 1st Gen AHSS TRIP steels, as well as several 3rd Gen AHSS grades like TRIP-Assisted Bainitic Ferrite, Carbide Free Bainite, and Quench & Partition Steels.

In these grades, increasing the stability of the retained austenite phase delays the austenite-to-martensite transformation to higher strain levels, further promoting formability improvements.

Several factors may promote higher RA stability, including additions of carbon (C) and manganese (Mn). Smaller austenite grains lower the martensite start temperature (Ms) and the number of martensite nucleation sites in each grain, and as such more energy (strain) is needed to start the transformation. 

Additions of silicon (Si), chromium (Cr), and aluminum (Al) are also beneficial to achieving the TRIP effect since each of these elements suppress cementite (iron carbide, Fe3C) formation and thereby allows for carbon enrichment of austenite.

However, Mn, Si, Cr, and Al all form oxides on the steel surface that hinder galvanizing and paintability associated with the e-coat layer.  Steelmakers typically choose an alloy development and processing strategy which minimizes the detrimental effects of these oxides.

Temperature also has an effect, not only from the paint-bake temperatures of approximately 170 °C, but from galvanizing at close to 500 °C.  Citation Z-20 studied the effects of temperature on 0.1%C-5%Mn Medium Manganese steels and found that while tensile strength was relatively independent with temperature, ductility slightly decreases as the temperature is raised from room temperature to 400 °C, but drops off substantially by 500 °C. To retain the formability benefits associated with RA grades, the article recommends galvanizing at temperatures below 400 °C.

In addition to the paint-bake and galvanizing temperatures, adiabatic heating from forming (including shearing and stamping) impact properties.  The temperature during forming can be influenced by the starting ambient temperature, the plastic energy dissipation, the latent heat of transformation and by conduction and convection to the environment.M-76

While deforming a metal, most of the energy is dissipated in the form of heat while only a small amount is stored. Austenite-to-martensite transformation kinetics are highly influenced by temperature, and the heating effects associated with mechanically-induced transformation can lead to a severe reduction in ductility.

The temperature rise due to dissipation is not negligible and since the TRIP effect is extremely sensitive to temperature, there is a need for a model to predict this behavior well.  Such a model is described in Citation M-76, which reviews that retained austenite stability is a function of several parameters such as temperature; carbon content and alloying elements; austenite grain size and morphology; austenite grain orientation and distribution within the microstructure; and hydrostatic pressure.

 

 

Back to the Top

 

 

Bake Hardenable

Bake Hardenable

AHSS, Conventional HSS, Steel Grades

BH Grades

Bake Hardenable (BH) steels grades are conventional High Strength Steels that exhibit a Bake Hardening Effect. BH steels exhibit an increase in yield strength after room-temperature stamping followed by processing through a thermal cycle comparable to the time-temperature profile used in paint curing (or baking) – approximately 170 °C for 20 minutes. Bake hardenability is characterized by determining the Bake Hardening Index.

Bake Hardenable steel grades have yield strength at shipment from the steel mills of 180 MPa to 300 MPa (approximately 25 ksi to 45 ksi). The grades at the lower strength levels are capable of being produced with a Class A surface finish and are used in applications where dent resistance is desired in thin sheet steel. Applications for the higher strength BH steels include structural parts where Class A surface is not required. The higher strength after forming and baking is the reason automakers might use these in body structure applications, potentially contributing to vehicle lightweighting efforts.

These grades work harden approximately 30 MPa when 2% strain is introduced, either from stamping or during a tensile test, which is similar to dent resistant IF-HS. In contrast to IF-HS, the paint-bake cycle after forming results in an additional yield strength increase. The minimum strength increase from baking is specified by some automakers as 20 MPa to 35 MPa, measured after applying a defined level of strain.

Higher yield strength directly improves the dent performance. Even though BH grades and their non bake hardening counterpart IF-HS grades may have similar yield strength and thickness after forming, bake hardenable steels will show superior dent resistance due to the increase in yield strength from the paint baking operation.

Ferrite is the main microstructural phase of BH steels. The strengthening from the paint bake cycle is due to the controlled amount of carbon remaining in solid solution (on the order of 25 ppm) when the steel leaves the production mill. At the baking temperatures after the part is formed, the dissolved carbon migrates to pin any free dislocations created from stamping. This increases the yield strength of the formed part for increased dent resistance. Formability does not suffer, since the strength increase occurs after stamping.

Most Advanced High Strength Steel (AHSS) grades also exhibit a Bake Hardening Effect, achieving yield strength increases of 40 MPa to 120 MPa from an appropriate thermal cycle. AHSS grades are not categorized with traditional bake hardenable steels, since their primary characteristics and applications are typically, but not exclusively, different. One exception are some Dual Phase (DP) steels available with a Class A surface, which are used as skin panels to combine excellent dent resistance with lightweighting benefits.

Some of the specifications describing uncoated Bake Hardenable (BH) steel are included below, with the grades typically listed in order of increasing minimum yield strength and ductility. Different specifications may exist which describe uncoated or coated, or steels of different strengths.

  • ASTM A1008M, with the terms BHS 26 [180], BHS 31 [210], BHS 35 [240], BHS 41 [280], BHS 44 [300]A-25
  • EN10268, with the terms HC180B, HC220B, HC260B, and HC300LAD-3
  • JIS G3135, with the term SPFC340HJ-3
  • JFS A2001, with the terms JSC270H, JSC340HJ-23
  • VDA239-100, with the terms CR180BH, CR210BH, CR240BH, and CR270BHV-3

 

Bake Hardening Effect

Bake Hardenable Steel Grades and most AHSS grades exhibit a Bake Hardening Effect, meaning that there is an increase in yield strength after room-temperature stamping followed by processing through a thermal cycle comparable to the time-temperature profile used in paint curing (or baking) – approximately 170 °C for 20 minutes.

The degree to which a sample is bake hardenable is characterized by the Bake Hardening Index.

In Bake Hardenable Steel Grades, solid solution hardening elements like phosphorus, manganese, and silicon are used to achieve the desired initial strength. For AHSS, the initial strength is determined by the balance and volume fraction of microstructural components like ferrite, bainite, retained austenite, and martensite. In both cases, a specifically engineered amount of dissolved carbon in the ferritic matrix causes an additional increase in the yield strength through controlled carbon aging during the paint-bake thermal cycle. The bake hardening process in AHSS grades is more complex, and results in substantially higher values of the Bake Hardening Index.

Figure 1 shows the work hardening and bake hardening increases for samples of three High-Strength steel grades having the same as-received yield strength prior to 2% pre-straining and baking. The HSLA steel shows little or no bake hardening, while AHSS such as DP and Transformation Induced Plasticity (TRIP) steels show a large positive bake hardening index. The DP steel also has significantly higher work hardening than HSLA or TRIP steel because of higher strain hardening at low strains. No aging behavior of AHSS has been observed due to storage of as-received coils or blanks over a significant length of time at normal room temperatures. Hence, significant mechanical property changes of shipped AHSS products during normal storage conditions are unlikely.

The higher bake hardening index (BHI) of AHSS grades DP 600 and TRIP 700 is also shown in Figure 2. While BHI is determined at a prestrain of 2%, this graph indicates that even higher levels of bake hardenability can be achieved with increasing strain. In a stamping where most areas have more than 2% strain, combining this higher bake hardenability with the increased work hardening that occurs with increasing strain results in a formed panel having a strength markedly higher than the incoming flat steel. This is beneficial for crash energy management.

Figure 1: Comparison of work hardening (WH) and bake hardening (BH) for TRIP, DP, and HSLA steels given a 2% prestrain. S1, K3

Figure 1: Comparison of work hardening (WH) and bake hardening (BH) for TRIP, DP, and HSLA steels given a 2% prestrain. S-1, K-3

 

Figure 2: Bake hardening responses of several HSS and AHSS products with varying pre-strain, reproduced from Figure 3 in Citation B-6. The bracketed numbers after each grade are references within the cited paper.

Figure 2: Bake hardening responses of several HSS and AHSS products with varying pre-strain, reproduced from Figure 3 in Citation B-6. The bracketed numbers after each grade are references within the cited paper.

 

Bake Hardenability of Exposed Quality Dual Phase Steels

Dent resistance is a function of the yield strength in the formed panel after it completes the paint baking cycle. Based on this premise, grades with higher bake hardenability, such as AHSS, should have substantially higher dent resistance. Application of AHSS grades to capitalize on improved dent resistance also requires their production at the desired thickness and width along with surface characteristics appropriate for Class A exposed quality panels. Some DP steels meet these tight requirements specified by the automotive industry.

A recent studyK-49 highlights this improved dent resistance. This work presents the experimental results and associated numerical investigation of the dent testing of DP270Y490T, a DP steel grade with 490 MPa minimum tensile strength. Tests performed to the SAE J2575 procedureS-7 measure the resultant dent depth after testing, so therefore smaller depths indicate improved performance. Compared with samples not processed through a bake hardening cycle, dent depth reductions occur with hotter and longer cycles, as shown in Figure 3. Increasing temperature plays a more significant role in dent depth reduction than increasing time. This work also reinforces that bake hardenability must be incorporated into simulation models in order to improve the accuracy of dent resistance predictions.

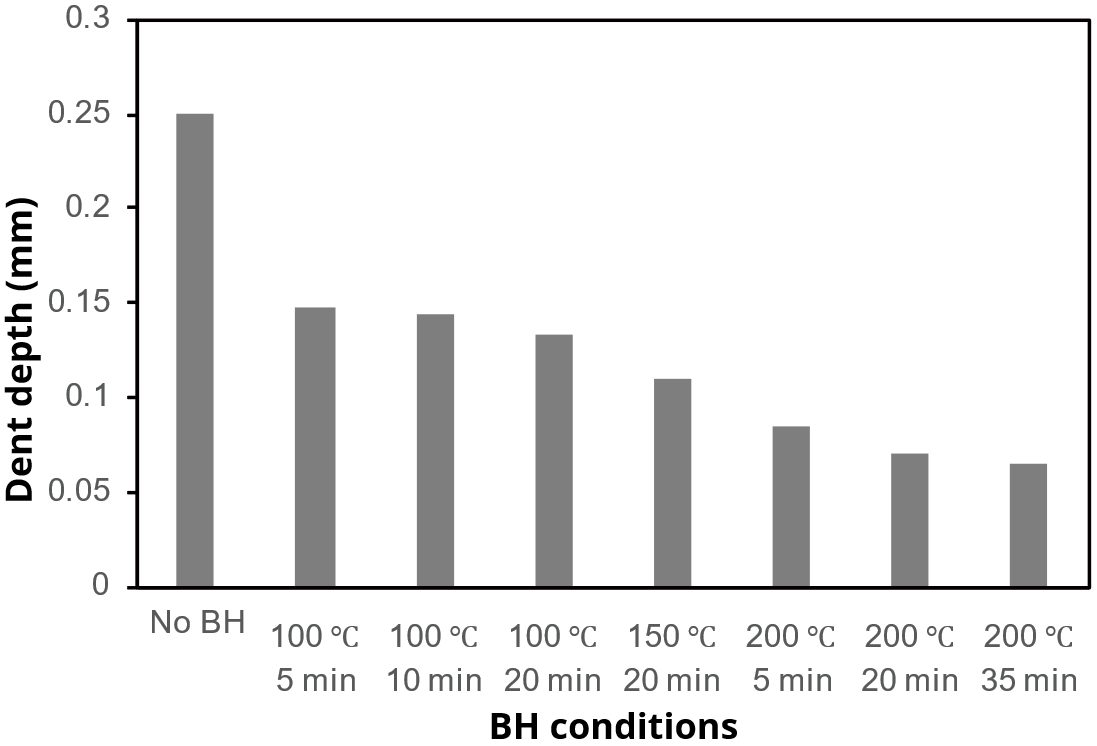
Figure 3: Dent resistance of DP270Y490T according to SAE J2575S-7* as a function of baking test conditions.K-49  Lower dent depth indicates better dent resistance.

Figure 3: Dent resistance of DP270Y490T according to SAE J2575S-7 as a function of baking test conditions.K-49 Lower dent depth indicates better dent resistance.

 

Bake Hardenability of 980 MPa and 1400 MPa Multi-Phase Steels with Different Prestrains

Citation X-5 evaluated the bake hardenability as a function of pre-strain on two steels having a microstructure of ferrite and martensite. The two steels are distinguished by their tensile strength:  Test steel 1# is a 980 MPa grade with a yield:tensile ratio of 80%, whereas test steel 2# is a 1400 MPa grade with a yield:tensile ratio of 85%.  See Table 1 for details.

Steel
ID #		 Thickness
(mm)		 Yield Strength
(MPa)		 Tensile Strength
(MPa)		 Elongation
(%)		 Elongation at Break
(%)
1# 1.5 832 1047 7 13.5
2# 1.4 1243 1455 4 7
Table 1: Mechanical Properties of Tested Steels. X-5

 

Conventional uniaxial tensile testing of a #5 JIS bar was used to apply prestrain and evaluate mechanical properties. After pre-straining, samples were tested by first bending coupons to 90°, followed by the conventional paint bake thermal cycle of 170 °C for 20 minutes except for the control samples which remained unbaked. In all cases, the bend line was parallel to the rolling direction.

In the absence of prestrain, no bake hardening was observed in the two steels, indicating that prestrain is a prerequisite for promoting bake hardening.

Increasing levels of prestrain did increase the bake hardening response, but only to a certain level, with the two steels having a different prestrain response. The lower strength Steel 1# reaches a maximum BH value of approximately 80 MPa at a prestrain of 2%, while the higher strength Steel 2# reaches a maximum BH value of approximately 160 MPa at a prestrain of 1%. See Figure 4.

Figure 4: Effect of prestrain on Bake Hardening of 980 MPa and 1400 MPa steels. X-5

Figure 4: Effect of prestrain on Bake Hardening of 980 MPa and 1400 MPa steels.X-5

 

The elongation to fracture response to increasing prestrain is found in Figure 5, which shows a continuous decrease in ductility.

Figure 5: Effect of prestrain on Elongation of 980 MPa and 1400 MPa steels. X-5

Figure 5: Effect of prestrain on Elongation of 980 MPa and 1400 MPa steels.X-5

 

Using these grades in production applications requires the formed product to be capable of withstanding additional damage if involved in a crash.  As such, some coupons bent to 90° were baked while others remained unbaked, and all were subsequently bent another 30°, producing a final bending angle of 60°. 

Baking has an adverse effect on the secondary bending performance of the 1400 MPa grade, which required a bend radius of 3 mm to avoid bend cracks in the baked condition. Without baking, a 2 mm radius was successfully bent.  In the 980 MPa grade, no cracking was found even with a 1 mm radius in either the unbaked and baked conditions.

 

Measuring The Bake Hardenability Index

Bake hardenability is characterized by determining the Bake Hardening Index, or BHI.

The Bake Hardening Index (BH2) is determined by taking a conventional tensile test sample and pulling it to 2% strain. This is known as a 2% pre-strain. The sample is then put into an oven for a thermal cycle designed to be typical of an automotive paint curing (paint baking) cycle: 170 °C for 20 minutes. The temperature and time may be different depending on the end-user specifications.

Some companies may specify BH0, which uses the same thermal cycle without the 2% pre-strain. BH5 or BH10 (5% or 10% pre-strain, respectively) may also be reported.

The experimental procedure and calculation of BH2 is standardized in EN 10325D-4 and JIS G 3135J-3, and is similarly described in several other specifications.

Figure 6 defines the measurement for work hardening (B minus A), unloading to C for baking, and reloading to yielding at D for measurement of bake hardening (D minus B).  Note that the bake hardening index shown here is measured up to the lower yield point, which is consistent with the EN 10325 definition.  JIS G 3135 prescribes the use of the upper yield point.

Figure 4: Measurement of work hardening index and bake hardening index.

Figure 6: Measurement of work hardening index and bake hardening index.  BHI is measured using the lower yield point in EN 10325D-4 and with the upper yield point in JIS G 3135J-3.

 

Back To Top

 

Bake Hardenable

Tensile Strength

Mechanical Properties

Engineering stress-strain units are based on the starting dimensions of the tensile test sample: Engineering stress is the load divided by the starting cross-sectional area, and engineering strain is the change in length relative to the starting gauge length (2 inches, 50mm, or 80mm for ASTM [ISO I], JIS [ISO III], or DIN [ISO II] tensile test samples, respectively.)

Metals get stronger with deformation through a process known as strain hardening or work hardening. This is represented on the stress strain curve by the parabolic shaped section after yielding.

Concurrent with the strengthening as the tensile test sample elongates is the reduction in the width and thickness of the test sample. This reduction is necessary to maintain consistency of volume of the test sample.

Initially the positive influence of the strengthening from work hardening is greater than the negative influence of the reduced cross-section, so the stress-strain curve has a positive slope. As the influence of the cross-section reduction begins to overpower the strengthening increase, the stress-strain curve slope approaches zero.

When the slope is zero, the maximum is reached on the vertical axis of strength. This point is known as the ultimate tensile strength, or simply the tensile strength. The strain at which this occurs is known as uniform elongation.

Strain concentration after uniform elongation results in the formation of diffuse necks and local necks and ultimately fracture.

Figure 1: Tensile Strength is the Strength at the Apex of the Engineering Stress – Engineering Strain Curve

Figure 1: Tensile Strength is the Strength at the Apex of the Engineering Stress – Engineering Strain Curve.