Forming Limit Curves (FLC)

topofpage

• Generating Forming Limit Curves from Equations
• Experimental Determination of Forming Limit Curves
• Differences between Forming Limit Curves and Forming Limit Diagrams

If all stampings looked like a tensile dogbone and all deformation was in uniaxial tension, then a tensile test would be sufficient to characterize the formability of that metal. Obviously, engineered stampings are much more complex.  Although a tensile test characterizes one specific strain path, a Forming Limit Curve (FLC) is necessary to have a map of strains indicating the onset of critical through-thickness necking for different linear strain paths. The strains which make up the FLC represent the limit of useful deformation. Calculations of safety margins are based on the FLC (Figure 1).

Figure 1: General graphical form of the Forming Limit Curve.^E-2

Picturing a blank covered with circles helps visualize strain paths.  After forming, the circles turn into ellipses, with the dimensions related to the major and minor strains. This forms the basis for Circle Grid Strain Analysis.

Generating Forming Limit Curves from Equations

Pioneering work by Keeler, Brazier, Goodwin and others contributed to the initial understanding of the shape of the Forming Limit Curve, with the classic equation for the lowest point on the FLC (termed FLC₀) based on sheet thickness and n-value. Dr. Stuart Keeler was the Technical Editor of these AHSS Guidelines through Version 6.0, released in 2017.

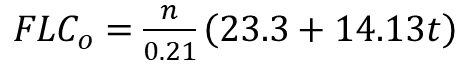Equation 1

These studies generated the left hand side of the FLC as a line of constant thinning in true strain space, while the right hand side has a slope of +0.6, at least through minor (engineering) strains of 20%.

Evidence accumulated over many decades show that this approach to defining the Forming Limit Curve is sufficient for many applications of mild steels, conventional high strength steels, and some lower strength AHSS grades like CR340Y/590T-DP. However, this basic method is insufficient when it comes to creating the Forming Limit Curves for most AHSS grades and every other sheet metal alloy.  Grades with significant amounts of retained austenite experience significant deviations from these simple estimates.^{H-23, S-61}  In these cases, the FLC must be experimentally determined.

Additional studies found correlation with other properties including total elongation, tensile strength, and r-value^{R-8, P-19, G-23, A-45, A-46, H-23} with some of these attempting to define the FLC by equations.

Experimental Determination of Forming Limit Curves

ASTM ^A-47 and ISO ^I-16 have published standards covering the creation of FLCs. Even within these standards, there are many nuances left for interpretation, primarily related to the precise definition of when a neck occurs and the associated limiting strains.

There are two steps in creating FLCs: Forming the samples and measuring the strains.

Forming sheet specimens of different widths uses either a hemispherical dome (Nakajima or Nakazima method ^N-14) or a flat-bottom punch (Marciniak method ^M-22) to generate different strain paths from which critical strains are determined. The two methods are not identical due to the different strain paths generated from the punch shape.  These differences may not be significant for many lower strength and conventional High-Strength Steels, but may deviate from each other at higher strengths or with advanced microstructures.  Figure 2 highlights samples formed with the Nakajima method.

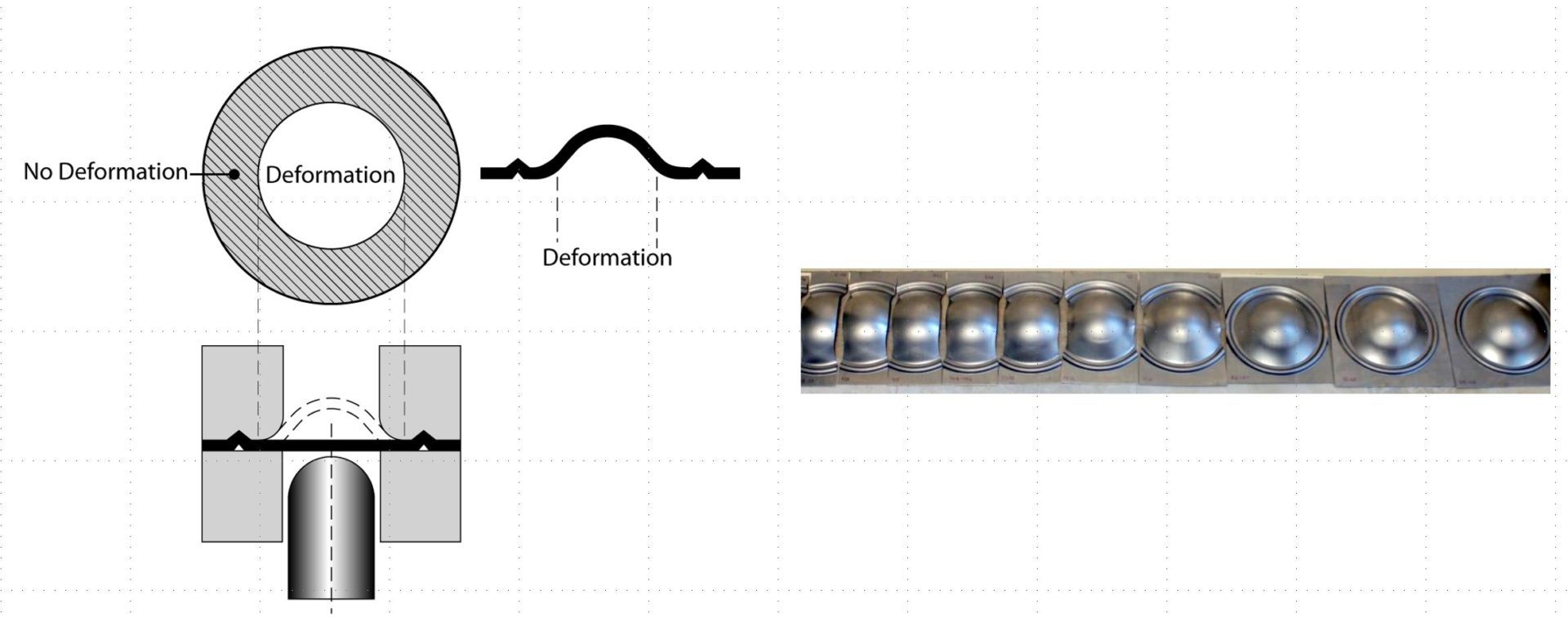
Figure 2:  Forming limit curves can be created by deforming multiple samples of different widths. Narrow strips on the left allow metal to flow in from the unconstrained edges, creating a draw deformation mode leading to strains that plot on the left side of the FLC.  Fully constrained samples, shown on the right^E-2, create a stretch deformation mode leading to strains that plot on the right side of the FLC.

Generally, strains are measured using one of two methods.  The first approach involves covering the initially flat test samples with a grid pattern of circles, squares, or dots of known diameter and spacing to measure the strains associated with deformation.  An alternate approach is based on Digital Image Correlation (DIC), where a camera tracks the movement of a random speckle pattern applied prior to forming.^{W-26, H-22, M-21}  DIC methods are directly suited for use with stress-based FLCs.

Differences between Forming Limit Curves

and Forming Limit Diagrams

Often, the terms Forming Limit Curve (FLC) and Forming Limit Diagram (FLD) are used interchangeably. Perhaps a better way of categorizing is to define the components and what they encompass.  This is somewhat of a simplification, since detailed interactions are known to occur.

• The Forming Limit Curve is a material parameter reflecting of the limiting strains resulting in necking failure as a function of strain path. It is a function of the metal grade, thickness, and sheet surface conditions, as well as the methods used during its creation (hemispherical/flat punch, test speed, temperature). It is applicable to any part shape.
• Deforming a flat sheet into an engineered stamping results in a formed surface with strains as a function of the forming conditions like local radii, lubrication, friction, and of course part geometry. These strains are essentially independent of the chosen metal grade and thickness.  Plotting these strains allows for a relative assessment of which strains are higher than others, but no judgment can be made on “how high is too high?”
• The Forming Limit Diagram is a combination of the Forming Limit Curve (a material property) and the strains (reflecting part geometry and forming conditions). The FLD provides guidance on which areas of the formed part requires additional attention to achieve robust stamping conditions.  Creating a subsequent FLD may be warranted when conditions change, since changes to the sheet metal properties (FLC) and the forming conditions (radii, lubrication, beads, blank size) will change the FLD, potentially affecting the conclusions.

Key Points

• • Conventional Forming Limit Curves characterize necking failure only. Fractures at cut edges and tight bends may occur at strains lower than that suggested by the Forming Limit Curve.
  • Differences in determination and interpretation of FLCs exist in different regions of the world. 
  • This system of FLCs commonly used for low strength and conventional HSLA is generally applicable to experimental FLCs obtained for DP steels for global formability.
  • The left side of the FLC (negative minor strains) is in good agreement with experimental data for DP and TRIP steels. The left side depicts a constant thinning strain as a forming limit.
  • Determination of FLCs for TRIP, MS, TWIP, and other special steels typically requires an experimental approach, since conventional simple equations do not accurately reflect the forming limits for these advanced microstructures.

Back to the Top

• Generating Forming Limit Curves from Equations
• Experimental Determination of Forming Limit Curves
• Differences between Forming Limit Curves and Forming Limit Diagrams