3rdGen AHSS, AHSS, Blog, Forming, main-blog, News, Press Hardened Steels, Roll Forming, Roll Stamping, Springback, Steel Grades
You’ll find this content as part of our page on Roll Forming, but this month, we want to highlight it in our AHSS Insights blog. Thanks to Brian Oxley, Product Manager, Shape Corporation, and Dr. Daniel Schaeffler, President, Engineering Quality Solutions, Inc., and Technical Editor – Metallurgy and Forming, AHSS Application Guidelines, for this case study.
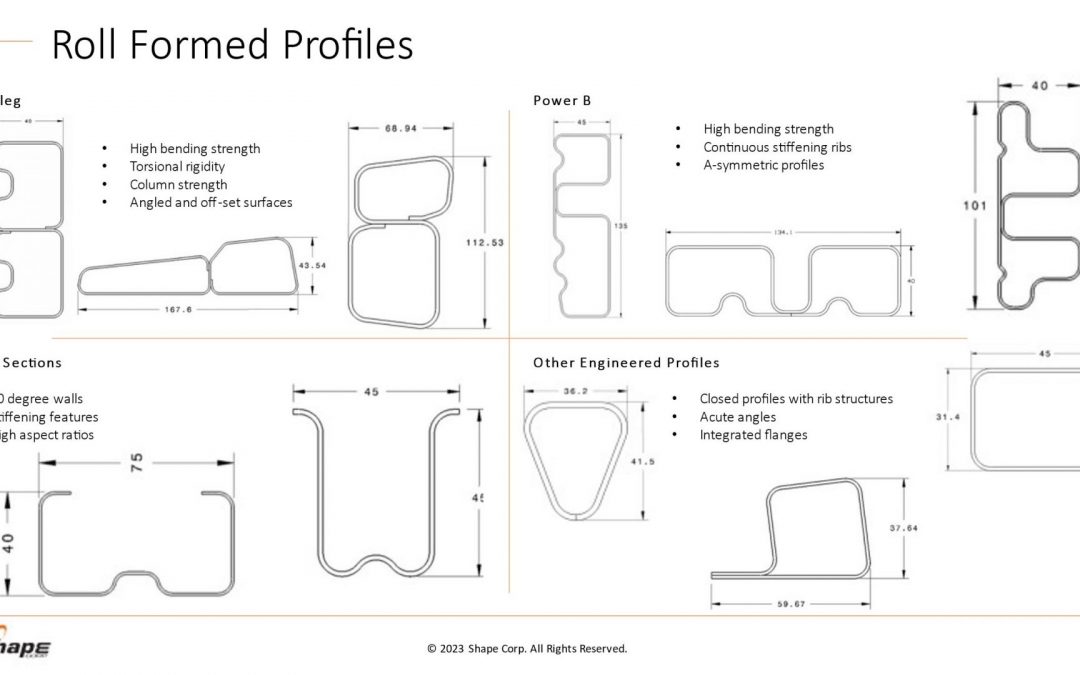
Roll forming is no longer limited to producing simple circular, oval, or rectangular profiles. Advanced cross sections, such as those shown in Figure 1, highlight some profile designs that aid in body structure stiffness and packaging space reductions.
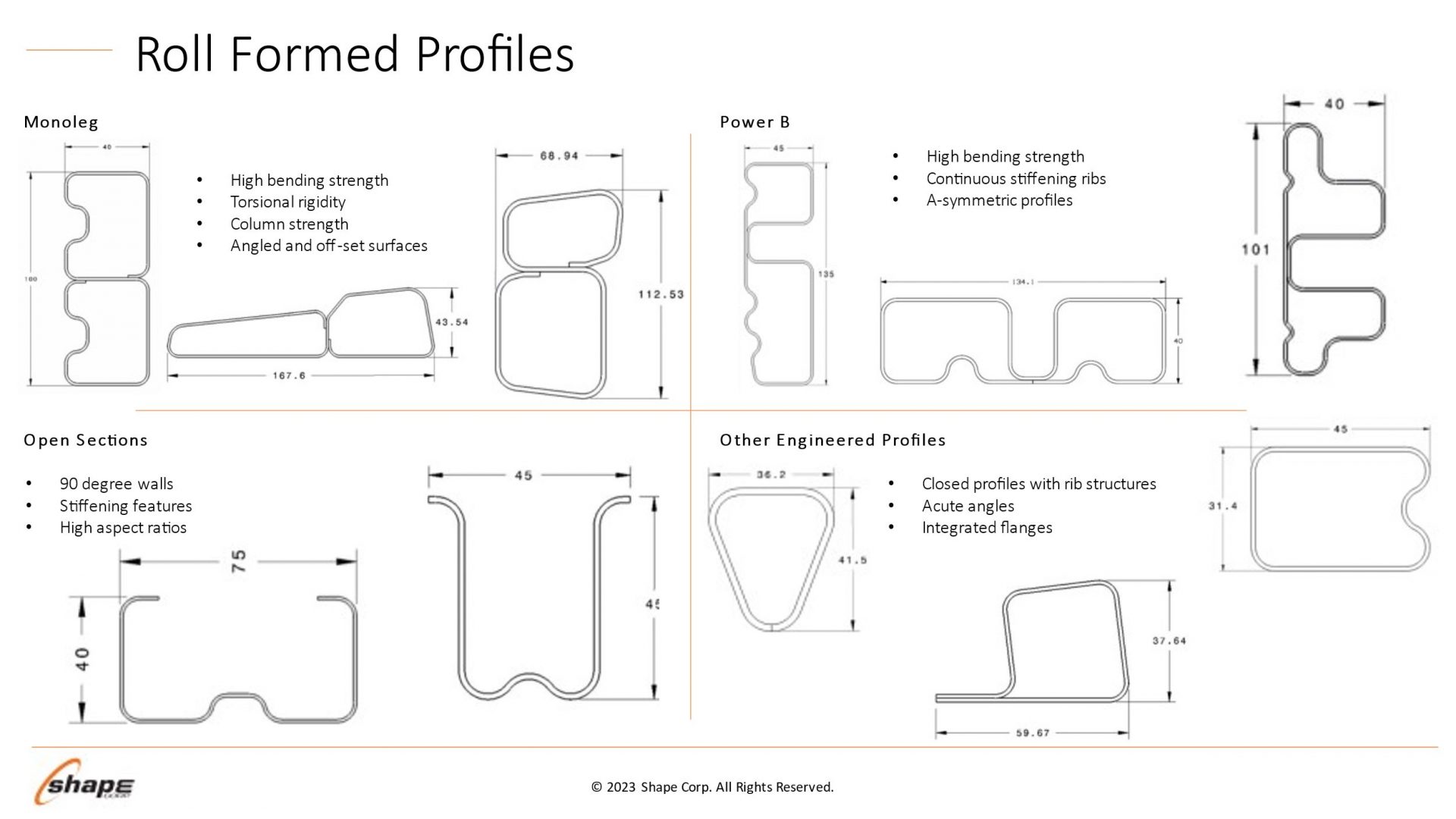
Figure 1 – Roll forming profile design possibilities. Courtesy of Shape Corporation.
Optimizing the use of roll forming requires understanding how the sheet metal behaves through the process. Making a bend in a roll-formed part occurs only when forming forces exceed the metal’s yield strength, causing plastic deformation to occur. Higher-strength sheet metals increase forming force requirements, leading to the need to have larger shaft diameters in the roll forming mill. Each pass must have greater overbend to compensate for the increasing springback associated with the higher strength.
Although a high-strength material requires greater forming loads, grades with higher yield strength can resist stretching of the strip edge and prevent longitudinal deformations such as twisting or bowing.
Force requirements for piercing operations are a function of the sheet tensile strength. High strains in the part design exceeding uniform elongation resulting from loads in excess of the tensile strength produce local necking, representing a structural weak point. However, assuming the design does not produce these high strains, the tensile strength has only an indirect influence on the roll forming characteristics.
Yield strength and flow stress are the most critical steel characteristics for roll forming dimensional control. Receiving metal with limited yield strength variability results in consistent part dimensions and stable locations for pre-pierced features.
Flow stress represents the strength after some amount of deformation and is therefore directly related to the degree of work hardening: starting at the same yield strength, a higher work hardening steel will have a higher flow stress at the same deformation.
Two grades are shown in Figure 2: ZE 550 and CR420Y780T-DP. ZE 550, represented by the red curve, is a recovery annealed grade made by Bilstein having a yield strength range of 550 to 625 MPa and a minimum tensile strength of 600 MPa, while CR420Y780T-DP, represented by the blue curve, is a conventional dual phase steel with a minimum yield strength of 420 MPa and a minimum tensile strength of 780 MPa. For the samples tested, ZE 550 has a yield strength of approximately 565 MPa, where that for CR420Y780T-DP is much lower at about 485 MPa. Due to the higher work hardening (n-value) of the DP steel, its flow stress at 5% strain is 775 MPa, while the flow stress for the HSLA grade at 5% strain is 620 MPa.
Figure 2 – Stress-strain curves for CR420Y780T-DP (blue) and ZE 550 (red). See text for description of the grades.
In conventional stamping operations, this work hardening is beneficial to delay the onset of necking. However, the use of dual-phase steels and other grades with high n-value can lead to dimensional issues in roll-formed parts. Flow stress in a given area is a function of the local strain. Each roll station induces additional strain on the overall part, and strains vary within the part and along the edge. This strength variation is responsible for differing springback and edge wave across a roll formed part.
Unlike conventional stamping, grades with a high yield/tensile ratio where the yield strength is close to the tensile strength are better suited to produce straight parts via roll forming.
Total elongation to fracture is the strain at which the steel breaks during tensile testing, and is a value commonly reported on certified metal property documents (cert sheets). As observed on the colloquially called “banana diagram”, elongation generally decreases as the strength of the steel increases.
For lower-strength steels, total elongation is a good indicator of a metal’s bendability. Bend severity is described by the r/t ratio or the ratio of the inner bend radius to the sheet thickness. The metal’s ability to withstand a given bend can be approximated by the tensile test elongation since, during a bend, the outermost fibers elongate like a tensile test.
In higher-strength steels where the phase balance between martensite, bainite, austenite, and ferrite plays a much larger role in developing strength and ductility than in other steels, microstructural uniformity usually limits bendability. Dual-phase steels, for example, have excellent uniform elongation and resistance to necking coming from the hardness difference between ferrite and martensite. However, this large hardness difference is also responsible for relatively poor edge stretchability and bendability. In roll-forming applications, those grades with a uniform microstructure will typically have superior performance. As an example, refer to Figure 2. The dual-phase steel shown in blue can be bent to a 2T radius before cracking, but the recovery annealed ZE 550 grade with noticeably higher yield strength and lower elongation can be bent to a ½T radius.
Remember that each roll-forming station only incrementally deforms the sheet, with subsequent stations working on a different region. Roll-formed parts do not need to use grades associated with high total elongation, especially since these typically have a bigger gap between yield and tensile strength.
We encourage you to visit https://ahssinsights.org/forming/roll-forming/roll-forming/ to learn more about roll forming and the types of coil shape that influence roll forming. Thank you to Brian Oxley and Dr. Daniel Schaeffler for providing this case study.

Brian Oxley, Product Manager, Shape Corporation, is a Product Manager in the Core Engineering team at Shape Corp. Shape Corp. is a global, full-service supplier of lightweight steel, aluminum, plastic, composite and hybrid engineered solutions for the automotive industry. Brian leads a team responsible for developing next generation products and materials in the upper body and closures space that complement Shape’s core competency in roll forming. Brian has a Bachelor of Science degree in Material Science and Engineering from Michigan State University.

Danny Schaeffler is the Metallurgy and Forming Technical Editor of the AHSS Applications Guidelines available from WorldAutoSteel. He is founder and President of Engineering Quality Solutions (EQS). Danny wrote the monthly “Science of Forming” and “Metal Matters” column for Metalforming Magazine, and provides seminars on sheet metal formability for Auto/Steel Partnership and the Precision Metalforming Association. He has written for Stamping Journal and The Fabricator, and has lectured at FabTech. Danny is passionate about training new and experienced employees at manufacturing companies about how sheet metal properties impact their forming success.
![Correcting Springback]()
Springback
Overview
Forming a part at room temperature creates elastic stresses, which will require some form of springback correction to bring the stamping back to part print. Forming at sufficiently high temperatures, such as with press hardening steels, allows for stress relief before the part leaves the die.
Springback correction can take many forms. The first approach is to apply an additional process that changes the elastic stresses to a less damaging form. One example is a post-stretch operation that reduces sidewall curl by changing the tensile-to-compressive elastic stress gradient through the thickness of the sidewall to all tensile elastic stresses though the thickness. Another example is over-forming panels and channels so that the release of elastic stresses brings the part dimensions back to part print instead of becoming undersized.
A second approach is to modify the process and/or tooling to reduce the level of elastic stresses created during the forming operation. An example is to reduce sidewall curl by replacing sheet metal flowing through draw beads and over a die radius with a simple 90 degree bending operation.
A third approach for correcting springback problems is to modify product design to resist the release of the elastic stresses. Here, mechanical stiffeners added to the part design lock in the elastic stresses and maintain desired part shape.
The approaches to springback correction described below are applicable to all higher strength steels, and typically will address both angular change and sidewall curl. AHSS grades having high flow stress in the formed part may require one or more of these approaches for satisfactory dimensional accuracy.
Minimizing springback through compensation in the first draw stage is more effective and less expensive than attempting to correct for existing springback in subsequent re-strike or re-forming operations. Approaches for improved dimensional accuracy include:
- Minimizing bending/unbending as the metal flows to the final part shape reduces distortion and tool wear.
- Reaching minimum strain levels across the panel minimizes springback and sidewall curl.
- Accounting for tool material loss during recuts helps ensure sufficient tool stiffness to counteract the higher press forces required with AHSS grades.
- Keeping the depth of AHSS channel-shaped parts as uniform as possible avoids forming distortions. Gradual shape transitions will minimize distortions, especially in areas of metal compression. Designing radii so there are only gradual transitions avoids stress risers and minimizes distortions. Minimize stretch/compression flanges wherever possible.
- If die and process design cannot relieve all elastic stresses, then creating a uniformly distributed residual stress pattern across the sheet and through the thickness will help eliminate the source of mechanical multiplier effects and thus lead to reduced springback problems.
Correcting Springback by Changing the Elastic Stress Distribution: Post-Stretch (Stake Beads, Hybrid Beads, and Active Binder Force Control)
One of the most effective techniques for significant reduction of both angular change and sidewall curl is a post-stretch operation. Applying an in-plane tensile force after all operations in which the sheet metal is bent and unbend over draw beads and across die radii can change the through-thickness tensile-to-compressive elastic stress gradients to all tensile elastic stresses.
When the part is still in the die, the outer surface of the bend over the punch radius is in tension (Point A in Figure 1), while at the same time the inner surface is in compression (B). After the punch retracts and the part is no longer under load, the tensile elastic stresses (A) tend to shrink the outer layers and the compressive elastic forces (B) tend to elongate the inner layers. These opposite forces form a mechanical advantage to magnify the angular change. The differential stress ∆σ is the driver for the dimensional change. Note that this reversal in stress direction after removal of the applied load is the same root cause that results in snap-through reverse tonnage reactions when punching higher strength steels.

Figure 1: Sheet metal bent over a punch radius has elastic stresses of the opposite sign creating a mechanical advantage to magnify angular change. Similar effects create sidewall curl for sheet metal pulled through draw beads and over die radii. When the part is no longer under load, it will change shape to relieve these elastic stresses.
In the case of side wall curl, this differential stress ∆σ increases as the sheet metal is work hardened going through draw beads and around the die radius into the wall of the part.
To correct this angular change and sidewall curl, apply a tensile stress to the flange end of the wall to generate a minimum tensile strain of 2% within the sidewall of the stamping. Figure 2 describes the sequence of events. The initial elastic states are tensile (A1) and compressive (B1). When approximately 2% tensile strain is added to A1, the strain point work hardens and moves up slightly to A2. However, when 2% tensile strain is added to B1, the compressive elastic stress state first decreases to zero, then climbs to a positive level and work hardens slightly to point B2. The neutral axis is moved out of the sheet metal. The stress differential ∆σ now approaches zero. Instead of bending or curving outward, the wall simply shortens by a small amount similar to releasing the load on a tensile test sample. This shortening of the wall length can be easily corrected by an increased punch stroke.

Figure 2: The large stress differential shown in Figure 1 is significantly reduced by applying a 2% tensile strain.
Post-Stretch with Stake Beads
Two die design methods currently in use can create the desired minimum 2% post-stretch on the sidewall of AHSS parts, both of which utilize what are commonly referred to as stake beads.
The first method involves retractable beads located in machined slots in the lower blankholder. The upper blankholder has machined stake bead pockets. Adjustable stop blocks located directly under the retractable stake beads can be shimmed to alter the timing when the stake bead engages the stamping, if required.
At the targeted punch stroke position, the retractable beads hit the stop blocks which forces them into the sheet metal flange. This creates a blank locking action while the punch continues to deform the part. As the die opens, the stake beads retract, and the cycle repeats itself from press stroke to press stroke. Adjustability of the draw bead height has advantages, particularly during initial die tryout.
As the retractable beads are inserts, removing them for hardening, coating, polishing, etc., is much easier than moving an entire die to perform bead work. Duplicate inserts can also be made, even utilizing alternative tool steels to increase durability. Locating the retractable stake bead on the blankholder, however, can lead to a larger required blank size. Die construction costs for retractable stake beads are also higher due to the additional machining required.
It is important to have adequate structural die support to avoid breaking the die. Significantly greater lateral thrust forces can cause catastrophic die failure if the stake beads are located too close to the punch opening. This is also true for many other die components, so die construction standards for mild and HSLA steels may not apply to AHSS.
An alternate approach is to locate the stake beads on the punch. Stake beads are machined directly in the punch casting, with the stake bead pockets machined into the upper die cavity. This approach may allow for a reduced blank size, since less material is needed outside the punch opening to accommodate both the draw bead and the stake bead. Figure 3 shows a DP590 B-pillar draw panel with draw beads located on the blankholder and stake beads located on the punch just inboard of the draw beads.

Figure 3: DP590 B-pillar with draw beads on the blankholder and stake beads on the punch. Yellow arrows point to the stake beads; blue arrows point to the draw beads located closer to the edge of the draw panel.
Figure 4 shows a draw die punch with stake beads machined at the very edge of the punch opening, along with the upper die with the stake bead pockets machined out.

Figure 4: Left Image – Stake beads machined into the punch of this DP780 die; Right Image: Stake bead pockets machined into the upper draw die. Note that there are no draw beads on this blankholder.
Draw beads control metal flow in draw dies. However, draw beads may become less functional when forming higher strength sheet steels having higher work hardening characteristics. Here, bending and straightening when pulling the sheet through the punch opening radius, combined with bearing on the binder surface, may be sufficient to control metal flow.
The punch and upper binder in Figure 4 have no draw beads, and instead exhibit stake beads that engage late in the press stroke. This solution is lower in cost but provides limited flexibility since the beads are machined directly into the die. In contrast, Figure 5 shows a die with removable stake bead inserts located on the punch. This approach has improved adjustability, but the added expense of machining the die and installing stake bead inserts.

Figure 5: This die has adjustable stake beads located on the punch as inserts, and do not retract during every press stroke. Note also that there are no draw beads on the blankholder.A-6
A third option to achieve the post forming 2% strain on the part with stake beads involves an additional die or die station. After nearly fully forming the part in the first die, a second die locks the remaining flange in place and further deforms the part by the additional 2%. This approach is expensive since it requires construction of an additional die, or if processed in a progressive die, adding this extra station increases the size and complexity of the progressive die.
Ensuring dimensional precision may require a restrike operation after trimming. In addition to sharpening the radii, the restrike die may provide the sidewall stretch (post-stretch) of approximately 2% required to eliminate curl.
Studies have shown that the height and geometry of the stake bead can impact springback control. Insufficient stretching below the targeted 2% post-stretch may not sufficiently address springback. If the restraining force of the stake bead is too great, it could lead to fracture at the bead or punch opening radius.
Adjusting the stake bead geometry around the part creates an unbalanced post-stretching configuration. Researchers applied this approach to counteract springback in a 3rd Generation steel, with more shallow beads used on deeper drawn sections of the tested part.J-11
The magnitude of springback is a function of the uniformity of the stress distribution through the thickness direction the wall of the formed sheet. An optimized combination of draw beads and stake beads promotes a uniform stress distribution through the thickness, which leads to improved springback control (Figures 6 and 9). Successful simulation provides a range of acceptable combinations of draw bead and stake bead heights.Z-15

Figure 6: A combination of draw beads and stake beads promote a uniform stress distribution through the thickness, leading to improved springback control.Z-15
Press Force and Energy Considerations when using Stake Beads
These post-stretch forming operations normally require significantly higher forming forces and energy requirements to be effective for several reasons.
Any sheet metal will work harden after going through draw beads. Since AHSS grades work harden to a greater degree than conventional high strength steels, the AHSS grades have significantly higher yield strength once the blank has passed over the draw beads. As stake beads are located inboard of draw beads, the stake bead must now deform material with a much higher yield strength due to the work hardening created by the bending and straightening of the material from being pulled through the draw bead.
Since stake beads engage late in the press stroke, their use has similar implications as creating embossments as discussed in a Press Energy case study in the Press Requirements page. This Case StudyH-3 shows that the last increment of punch travel required to finish embossing requires significantly higher energy for AHSS versus conventional mild or HSLA steel. In the current discussion on stake beads, inducing an additional 2% post forming strain into the part will also require higher forming forces and energy requirements, since the forming process work hardened and strengthened the sidewalls. Even if the press has sufficient force and energy characteristics, avoid necking down and tearing the sheet metal as it bends over the punch radius.
Case Studies: Using Stake Beads to Reduce Springback L-1
This case study evaluated springback on specially constructed dies tested using several grades of conventional and advanced high strength steels, including HSLA, dual phase, and TRIP grades with tensile strength ranging from 450 MPa to 980 MPa. One die used the conventional approach, allowing for metal to flow from the flange without a bead. The second die had a recessed square stake bead in the flange that created a post-stretch condition near the end of the stroke. As expected, the post-stretch die resulted in dramatically lower springback (Figure 7). In addition, springback on parts made with this die was not a function of the sheet metal tensile strength, making for a more robust process.

Figure 7: Comparison of two DP 450/750 parts, where stake beads in the bottom part minimized springback. Both images are different angles of the same parts.L-1
Post-Stretch with Hybrid Beads
As seen in the prior figures, the stake beads geometry plays an important role in restricting the metal flow when stretching the sidewalls during the last stages of the punch stroke. This approach requires a larger blank to accommodate the bead, and must be used in a press with sufficient tonnage to set the bead.
A modified approach significantly reduces the blank size requirements as well as lowers the required tonnages to create the bead. It uses stinger beads to penetrate the sheet metal as well as create a wave shape in the deformed region which restricts metal flow. This so-called hybrid bead takes up less than 25% of the bead surface area compared with a conventional stake bead, allowing for a measurably smaller blank. There is no bending over tight bead radii, eliminating the splitting risk at the bead. The restricted metal motion across the beads further minimizes the stress differential between the two sheet steel surfaces, which eliminates a significant root cause of sidewall curl.
Figure 8 highlights the influence a hybrid bead has on springback of a 3rd Generation Steel having a minimum tensile strength of 980MPa.

Figure 8: Hybrid bead dramatically reduces springback in a 3rd Generation steel having 980 MPa minimum tensile strength, while taking up less space and requiring reduced tonnage than stake beads.A-6
Animations of the process sequence is presented in Figures 9 and 10, with more information available in Citations J-12 and W-19.
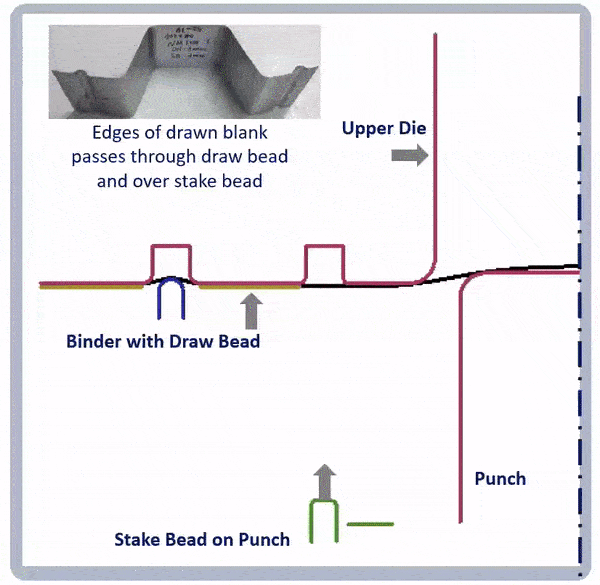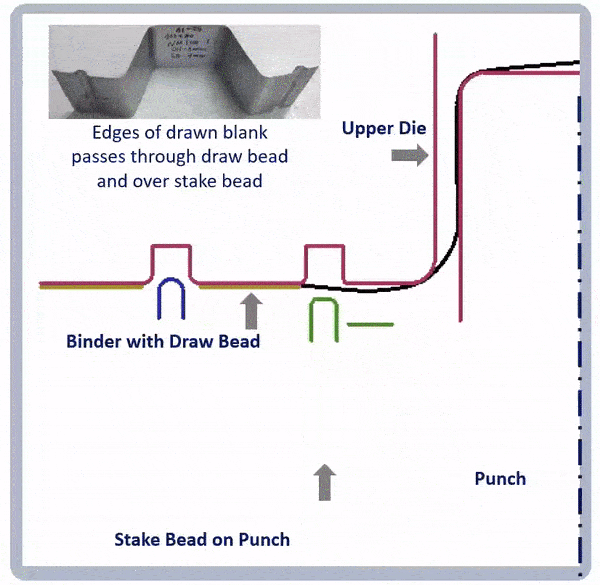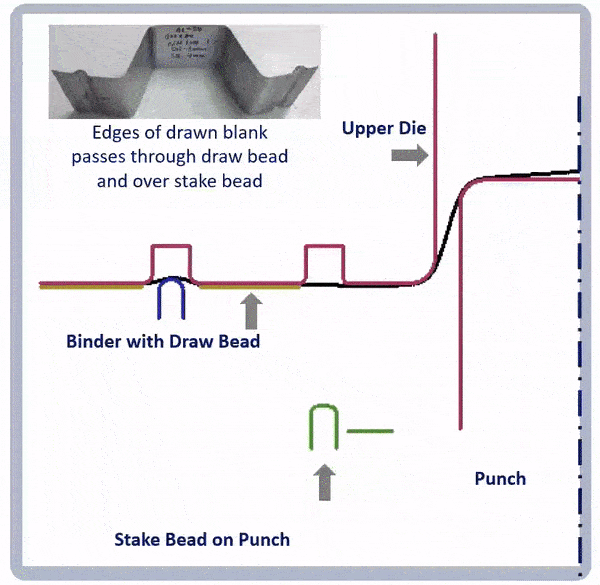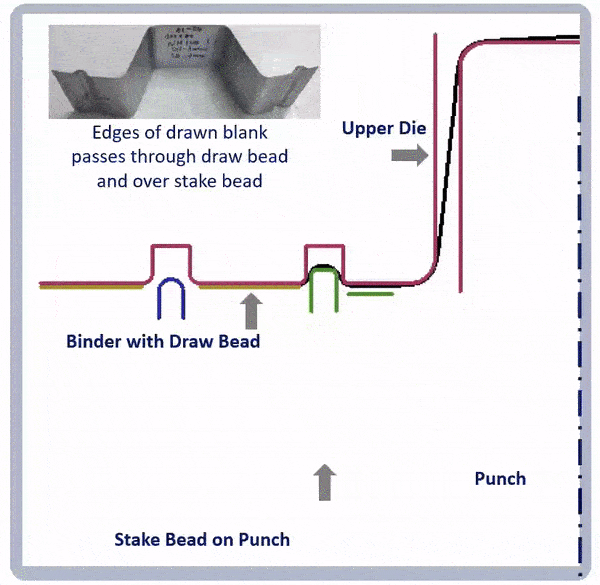
Figure 9: Animation showing a combination of draw beads and stake beads to reduce springback.A-6
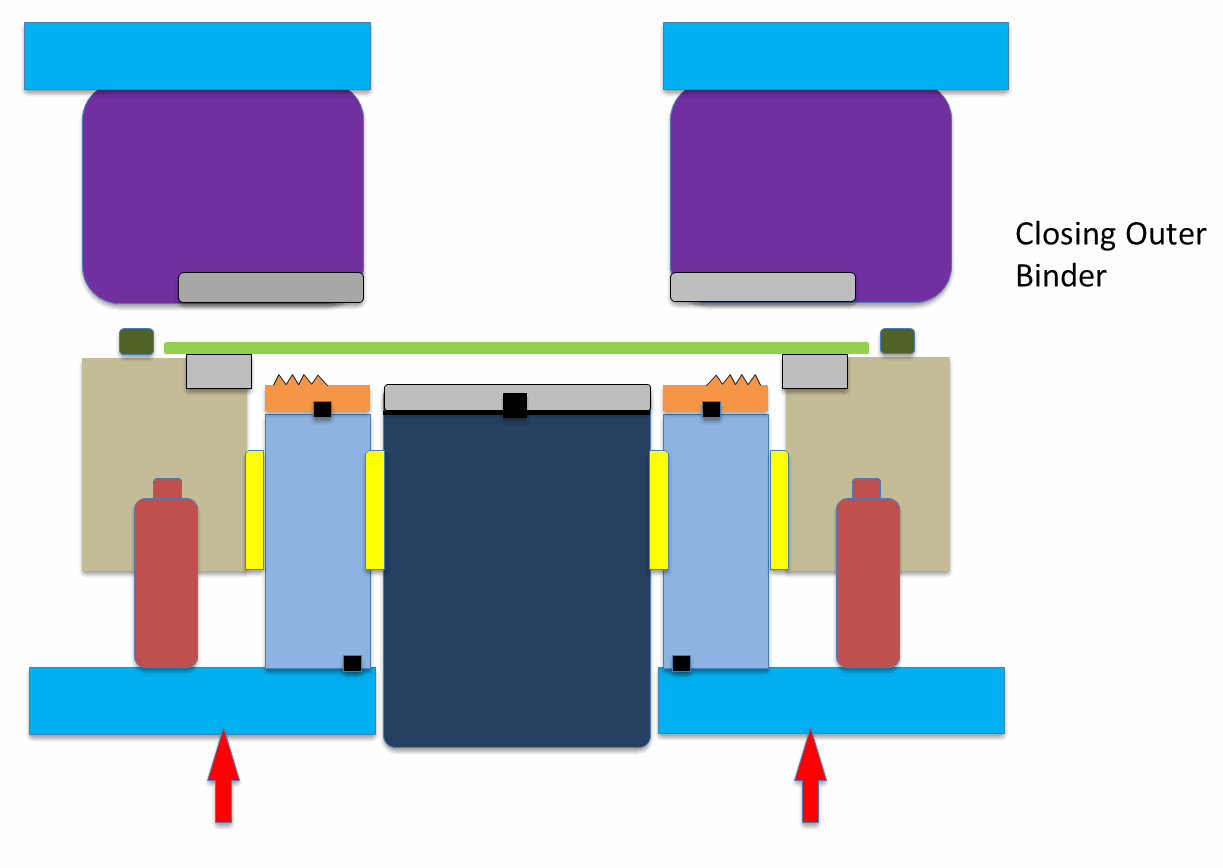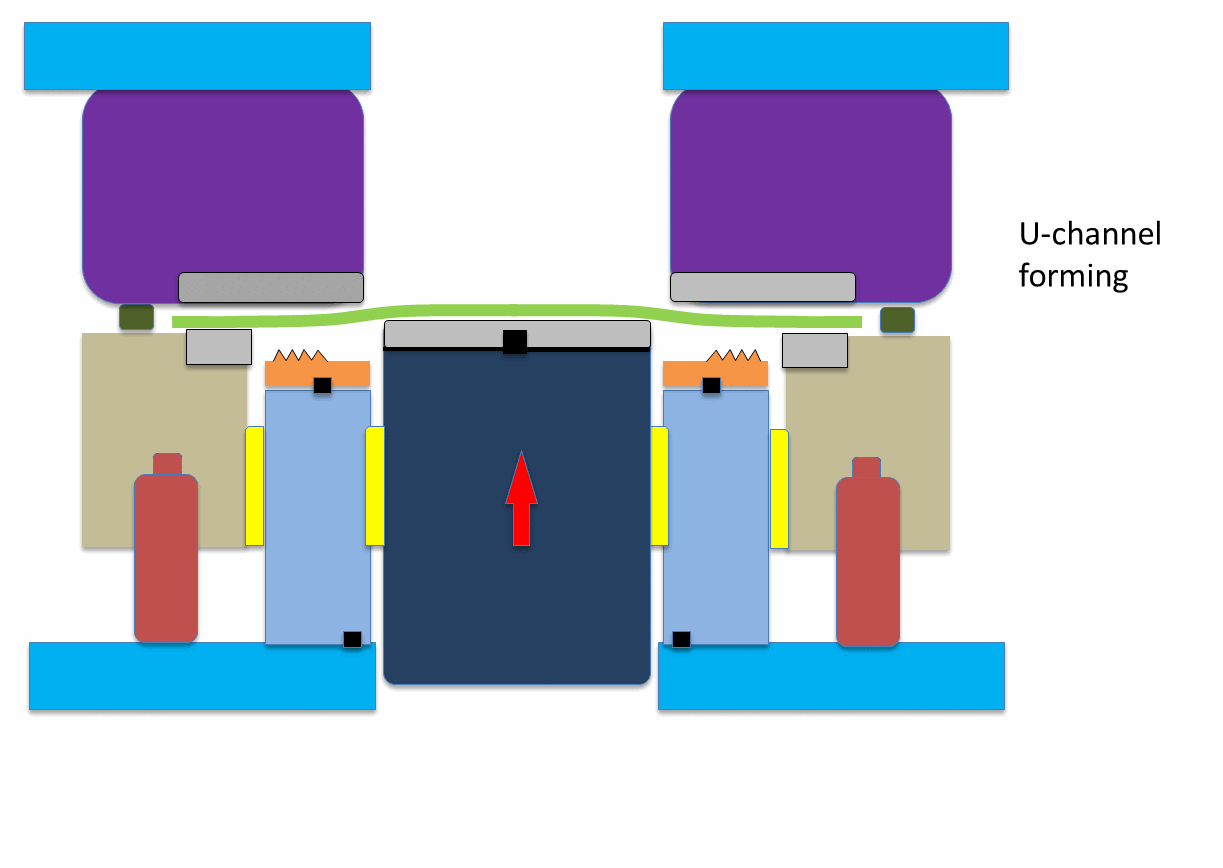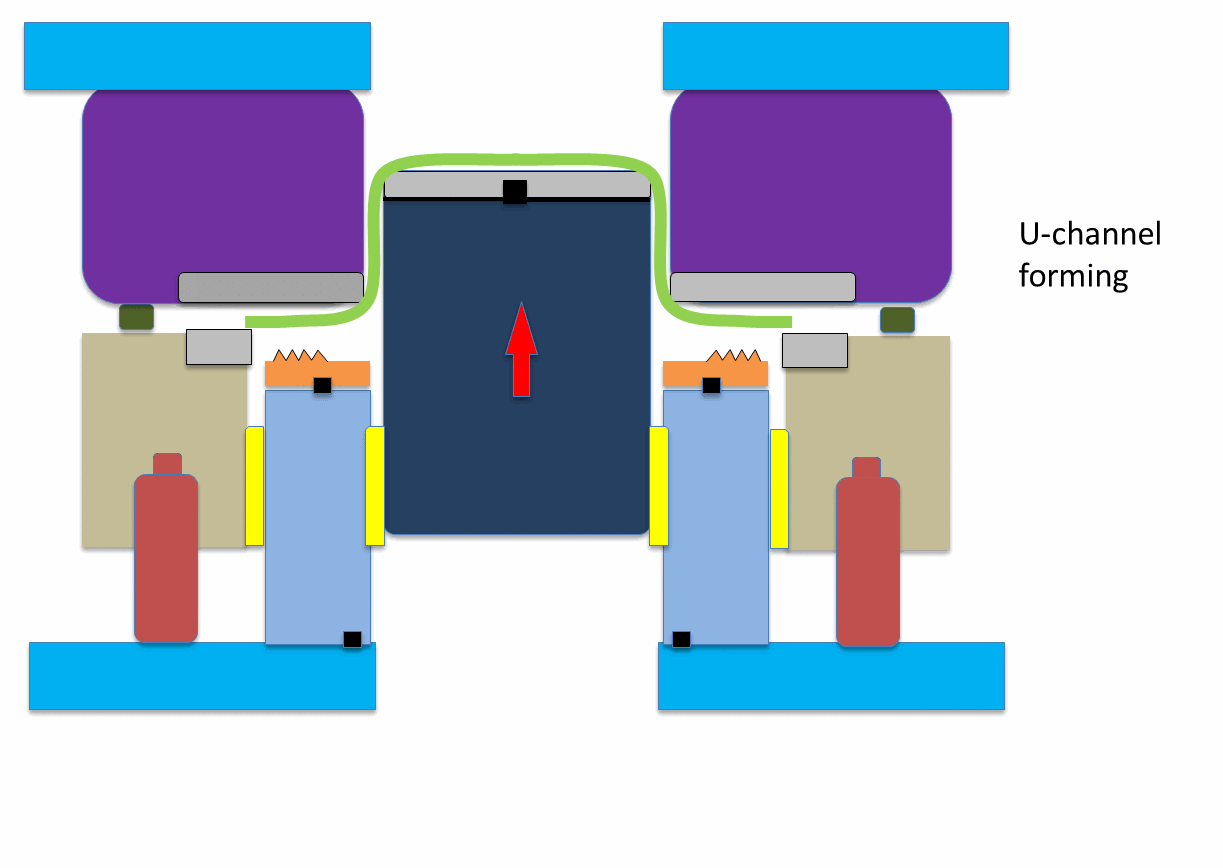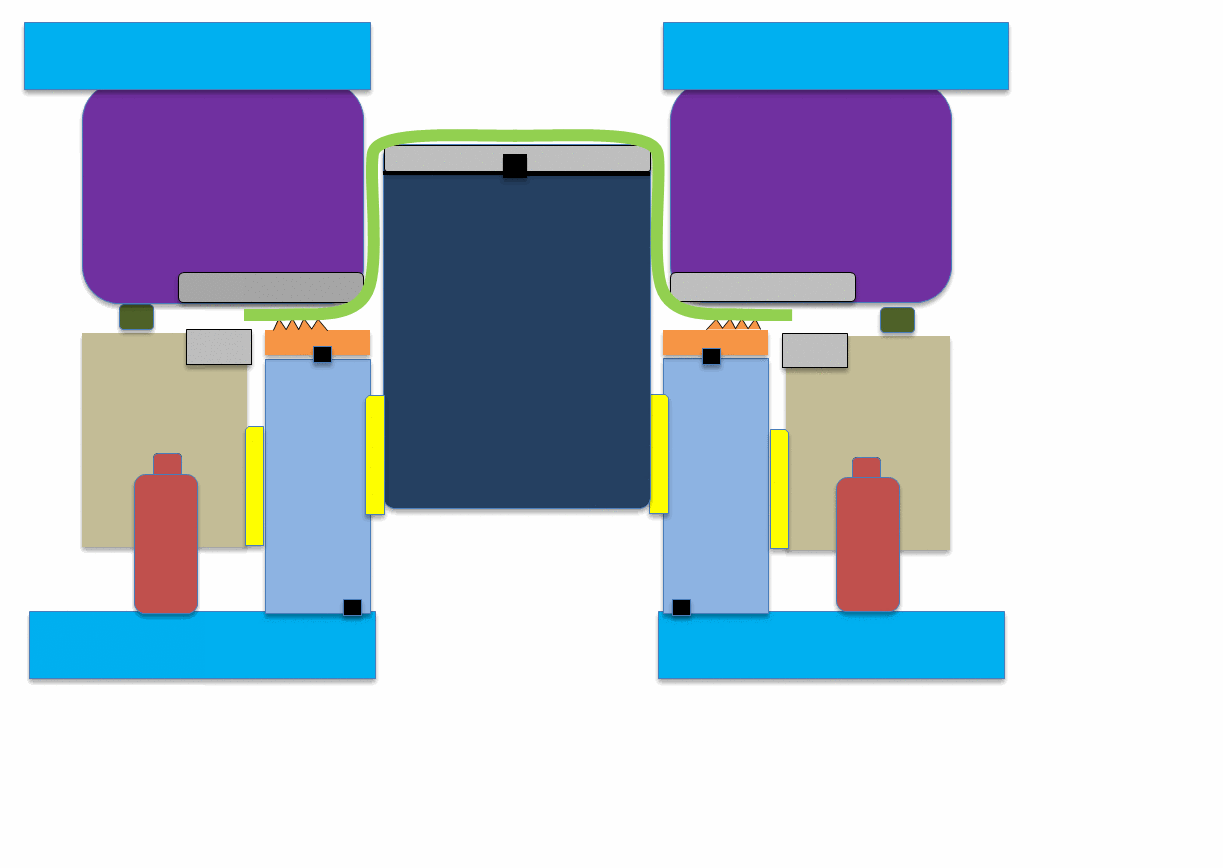
Figure 10: Animation showing application of hybrid beads to reduce springback.A-6
Post-Stretch with Active Binder Force Control
While most stamping processes apply binder pressure uniformly throughout the press stroke, modern stamping presses can be equipped with cushions having multipoint-control systems. Adjusting the associated pressure profile around the panel and throughout the stroke optimizes metal flow, prevent splits and wrinkles, and minimize thinning. This active binder force control capability allows for application of increased blank holder force at any point of the stroke, including at the end to achieve the targeted 2% post-stretch needed to minimize springback. (See the AHSS Insights blog by Dr. Daniel Schaeffler from Engineering Quality Solutions, Inc.)
Beyond simply increasing the blank holder force at the end of the stroke, varying the pressure sequence during the stroke may be beneficial as well. DP 340/590 steel was stamped using a constant binder force, an increasing binder force starting low and finishing high, and a third approach where the initial part of the stroke formed the part with a medium binder force which dropped for most of the stroke but increased at the end to apply the sidewall tension. This third approach resulted in the least angular change and sidewall curl, as seen in Figure 11.

Figure 11: DP 340/590 stamped with constant binder force (top), low-to-high binder force (middle), and medium-to-low-to-high binder force (bottom).P-14
Many angular change problems initiate when constructing the tooling either to part print or without sufficient springback compensation. Achieving targeted dimensions may require over-forming or over-bending.
Use rotary bending tooling where possible instead of flange wipe dies. Rotary bending allows for easy adjustment of the bending angle to correct for changes in springback due to variations in steel properties, die set, lubrication, and other process parameters. In addition, the tensile loading generated by the wiping shoe is absent.
However, if over-bending with flange wipe dies is the chosen approach to minimize angular change, use die radii less than the part radius and use back relief for the die and punch (Figure 12). This approach intentionally subjects the bending radius to compressive stresses between the punch and die. The squeezing and associated thinning at the bend radius results in plastic deformation of the sheet steel, with little elastic recovery after unloading from the press.

Figure 12: Providing back relief on the flange steel and lower die aids over-bending.A-2
Cross section design for longitudinal rails, pillars, and cross members impacts the effectiveness of springback compensation methods. The rail cross section in sketch A of Figure 13 does not allow the use of over-bending for springback compensation in the forming die. In addition, this design likely leads to severe sidewall curl in AHSS channel-shaped cross sections. Minimize these quality issues by designing a cross section that allows for over-bending during forming, as shown in sketch B. Reduced sidewall curl is another benefit of this cross-sectional design. Springback allowance must increase as strength increases. Typical wall opening angles are 3-degrees for Mild steel, 6 degrees for DP 350/600 and 10 degrees for DP 850/1000 or TRIP 450/800. In addition, the cross section in sketch B will have the effect of reducing the impact shock load when the draw punch contacts the AHSS sheet. The vertical draw walls shown in sketch A require higher binder pressures and higher punch forces to maintain process control.

Figure 13: Changing a rail cross section from A to B allows easier over-bending for springback control.N-3
Producing closed box sections involves welding two channel sections together at their flanges. Hat-sections with 90-degree corners such as seen in sketch A of Figure 14 will experience many production problems since each component will have issues with sidewall curl and angular change. The hexagonal section in sketch B will reduce sidewall curl and twist problems, while permitting over-bend for springback compensation in the stamping dies.

Figure 14: Changing the design of a closed cross section from A to B leads to a reduction in springback problems.N-3
Adding extra stages to the forming process allows for secondary operations to return a sprung part back to nominal dimensions. For example, Figure 15 shows a process where a crown existing in the first step when forming a channel section uses a second die for flattening and eliminating sidewall springback.

Figure 15: Schematic showing how flattening a crown corrects angular springback.A-3
As a related process, multiple stage forming (Figure 16) is an option to minimize springback and improve dimensional accuracy when stamping rails or other parts with a hat-shaped cross section consisting of right angles. This processing approach creates a design which avoids re-working previously-formed (and therefore work hardened) sections.
In the first operation, all 90-degree radii and mating surfaces are formed using “gull-wing” processes with over bending to compensate for springback. The larger radius in the top of the hat section gets flattened in the second stage. Certain cases may require an over bending of the flat top section.
Multiple-stage forming also helps when forming parts having small geometrical features of severe geometry that can be formed only in the re-strike operation. A part that has a variable cross section in combination with small geometrical features may need a coining operation in the second or last stage of the forming process. This may be the only way to control the geometry.

Figure 16: Two-stage forming produces a dimensionally accurate hat section with relatively small radii.R-1
Correcting Springback by Reducing or Minimizing the Elastic Stresses
The process design, and therefore the tooling design, can drastically affect the level of the elastic stresses in the part.
As an example, different blankholder actions provide four possible processes to form a hat-profile channel, each with different dimensional accuracy (Figure 17). The four processes are:
- Draw: the conventional forming type with continuous blankholder force and all blank material undergoing maximum bending and unbending over the die radius. This forming mode creates maximum sidewall curl.
- Form-draw: a forming process in which application of the blank holder force occurs between the middle and last stage of forming. It is most effective to reduce the sidewall curl because this approach minimizes bend unbend deformation at the beginning of the stroke prior to application of any blank holder force. Applying a large tensile stress during the last stage of forming creates the post-stretch condition to further minimize curl.
- Form: a process where the flange is created during in the last stage of forming and the material undergoes only a slight amount of bend-unbend deformation. Depending on the part geometry, the lack of a blankholder may lead to wrinkles.
- Bend is a simple bending process to reduce the sidewall curl, which avoids the bend and unbend sequence associated with sidewall curl. However, this approach likely leads to an angular change in the sidewall.

Figure 17: Four processes for generating a channel for bumper reinforcement create different levels of elastic stress and springback.K-5
Correcting Springback by Locking in the Elastic Stresses
Where part design allows, geometrical features like darts, beads, and stiffeners prevent the release of the elastic stresses and reduce various forms of springback. Minimize twist by adding strategically placed vertical beads, darts, or other geometric stiffeners in the shorter length wall to equalize the length of line.
Applying these features in a restrike operation may not be possible due to equipment or design limitations, since the yield strength of the sheet metal increases after work hardening. In the case of AHSS grades, this work hardening leads to a dramatic increase in strength.
Any elastic stress locked into the panel remains in the part as residual or trapped stresses. Subsequent forming, trimming, punching, heating, or other processes may unbalance the residual stress and change the part shape. For these reasons, the full process needs to be simulated, incorporating both material and geometrical changes occurring in all prior operations.
Figures 18 to 22 present examples of how elastic stresses can be locked into the part to control for springback.

Figure 18: Geometrical stiffeners like flanges or beads lock elastic stresses into the part, fixing the part shape.A-2

Figure 19: B-pillar using stiffening darts to control springback.A-41

Figure 20: Step flange locks in elastic stresses on a draw wall.A-41

Figure 21: Hat section showing the effects geometrical features have on controlling springback. Angular change and sidewall curl are noticeably less pronounced on the left side having the vertical beads, compared with the right side where no springback mitigation methods were employed.K-14

Figure 22: DP600 front rail upper reinforcement with vertical beads designed into the part geometry for springback control.F-9
An American Iron and Steel Institute study defined several tool and process parameters that reduced angular change and side wall curl (Figures 23 and 24). As expected, angular change and curl increase with yield strength. Tighter clearances, smaller punch radii, and higher drawbead restraining forces reduced both types of springback.S-47

Figure 23: The effect of tool parameters in angular change. The lower values are better.S-47

Figure 24: The effect of tool parameters in sidewall curl. Higher values of radius of curl are better.S-47
Figure 25 highlights the importance of keeping the die clearance as tight as allowed by formability and press capability. Unwanted bending and unbending work hardens the sheet metal and promotes increases in springback.

Figure 25: Reducing die clearance restricts additional bending and unbending as the sheet metal comes off the die radius, minimizing angular change.Y-2
Figure 26 illustrates that angular change increases dramatically for higher strength steels as the bend radius increases. Minimizing angular change requires designing the punch radius as sharp as formability and product/style allows. However, with AHSS grades, sharp radii may promote the local formability failure mode of shear fracture. Inside product feature radii should be a minimum of 3T for any AHSS grade having a tensile strength at or above 590 MPa. Die radii at draw beads, punch openings, etc., where the material is pulled under tension over a radius should be at least 5T for any AHSS grade over 590 MPa. Minimum bend radii requirements may be even greater as strength levels continue to increase, roll forming excluded. To avoid problems due to specifying the wrong AHSS grade for the wrong application, bend testing and hole expansion testing should be used in conjunction with close communication with the steel supplier when selecting the proper die radius for the intended AHSS material. In addition, sharp radii contribute to excessive thinning at the tangent to the radius when global formability failures are of concern.

Figure 26: Angular change increases with yield strength and bend radius at a constant thickness.S-2
Case Study in Springback Reduction Strategies
Automakers face conflicting constraints of lightweighting while improving safety performance. The B-Pillar Inner plays a significant role in meeting ever-increasing roof crush and side impact performance requirements. A studyM-17 published in 2007 showed the tooling and design changes made when transitioning from HSLA 340/440 to DP 550/980. These changes, shown in Figure 27, include adding sidewall beads to control springback, along with geometry to improve section stiffness.

Figure 27: Strategies to reduce springback in a DP 550/980 B-Pillar Inner.M-17
Another approach is highlighted in a Webinar from 2020.S-105 When forming hat-shaped sections, sidewall curl is minimized by using a cam to form the sidewall-flange area, followed by a more conventional sidewall stretch at the end (Figure 28). The animation in Figure 29 shows the process.S-6

Figure 28: Cam bending of sidewall-flange area minimizes springback.S-105
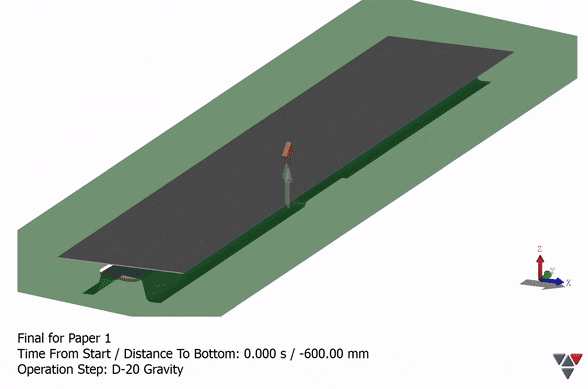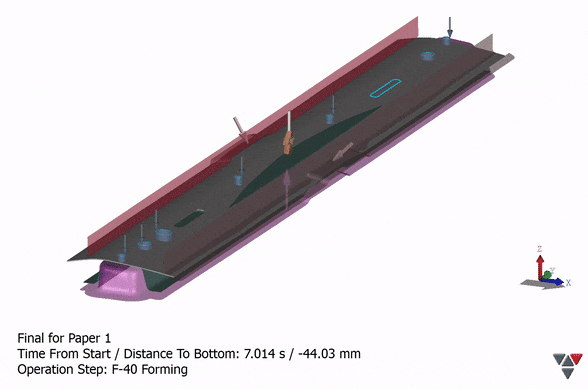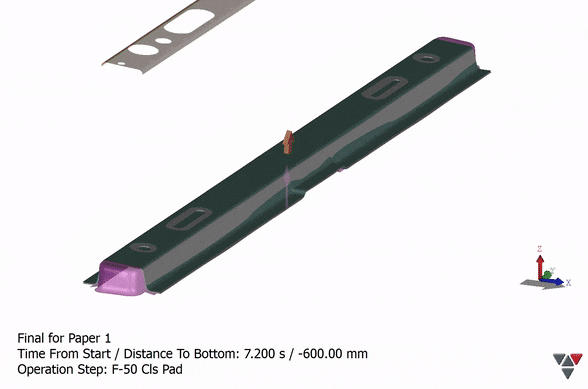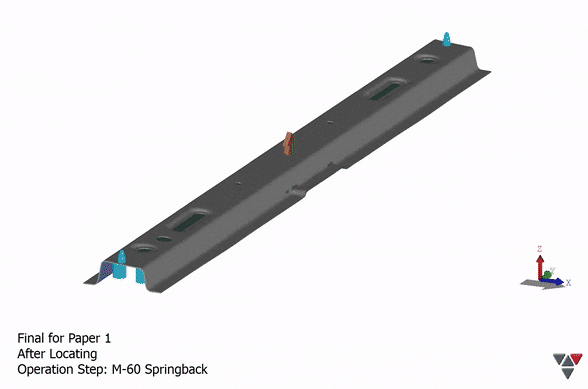
Figure 29: Animation of hat-section forming process to minimize springback.S-6
Compressive Stress Superposition for Springback Reduction
As explained above, springback and curl initiate when there are tensile stresses on one surface and compressive stresses on the other surface of the sheet. One of the keys to springback reduction is balancing these stresses through the sheet thickness.
A patented process known commercially as Smartform® relies on compressive stress superposition to achieve this stress balance. B-76 , L-65, B-77,, V-23
Achieving compressive stress superposition uses a two-step process where in the first preform step, the blank is formed into a “U” shape having a similar contour to the finished component, and then a sizing step to adjust the dimensional accuracy. This second step compresses the sheet metal during sizing rather than making it thinner from a drawing operation.
In the preforming stage (OP20), geometrical flexibility in the design of the tool surface exists, as the final component contour is not set until the sizing calibration stage (OP30). This allows for enlarged radii and wall angles to be used as countermeasures to prevent wrinkling and cracks.
Since the preform is not drawn, the starting blank can be cut to almost the same size as its final geometry before it is formed, reducing the number of trimming operations in the die process, as well as the amount of material used. Reportedly, an average of 15 percent materials savings occurs relative to conventional types of forming, with the actual savings being dependent on the size and complexity of the component.
Another benefit: resulting from the way the stress balance is achieved, this technology is relatively insensitive to variations in sheet metal properties, leading to a highly robust process.
Compressive stress superposition has been shown to work for steels having minimum specified tensile strength of 590 MPa through 1180 MPa.
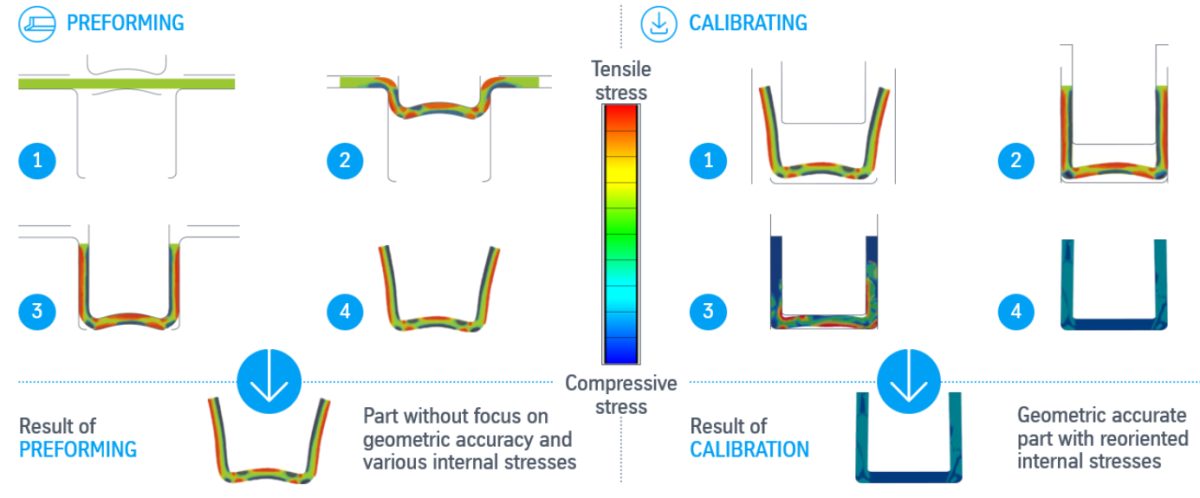
Figure 30: Compressive Stress Superposition Stress Conditions. Left: Preforming (OP20). Right: Sizing and Calibration (OP30). L-65
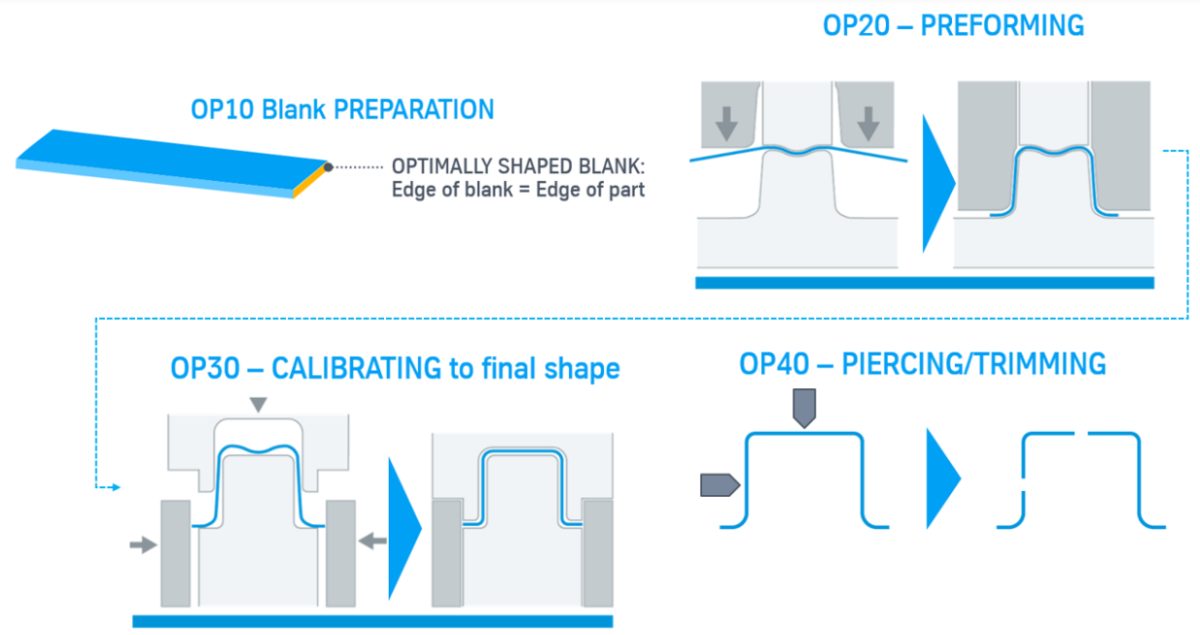
Figure 31: Compressive Stress Superposition Die Process.L-65
Key Points
Several process and design modifications remove (or at least minimize and stabilize) the different modes of springback found in channels and similar configurations:
- Open wall angles achieved with minimized metal flow over die radii minimize angular change and sidewall curl.
- Overbending accounts for angular change, allowing the part to spring back to the targeted dimensions. Subjecting the bending radius to compressive stresses at the bottom of the press stroke plastically deforms the sheet metal, minimizing the elastic stresses associated with springback. Note that this approach is less successful in addressing sidewall curl.
- Applying in-plane tension to the side walls after forming reduces springback. Methods include using stake-beads, hybrid beads, or active binder force control. However, with higher strength steels, it may be difficult to achieve sufficient stretch to the sidewall by simply increasing the blank holding force. Preventing metal flow into the wall during post-stretching may require lock beads. Bead geometry affects blank size and press requirements.
- Geometric stiffeners like darts, beads, and flanges lock elastic stresses in place preventing springback from occurring. Subsequent forming or trimming operations may relieve these stresses, resulting in dimensional distortion. In addition, the selected press must have the load and energy requirements to form these features.
Design the part and tool in such a way that springback is desensitized to variations in material, gauge, tools and forming processes (a robust system and process) and that the effects of springback are minimized rather than attempt to compensate for it.
The accuracy of forming simulation programs to predict springback continues to improve, especially if it incorporates a complete material characterization and the chosen model is appropriate for the challenges of the specific part and forming method. Even without full accuracy, springback simulations can predict the trend and test the effectiveness of proposed countermeasures. In all cases, verify predictions against physical measurements.
Back To Top
![Correcting Springback]()
Forming, Springback
Decades ago, the major concern in sheet metal forming was elimination of necks and tears. These forming problems are a function of plastic strain, and addressing them involves maintaining strain levels in the part below specific critical strains. Forming limit diagrams, which combine the formability of the steel with the part shape and forming process, show where these critical areas fall out on the part. In-service structural requirements may result in additional limitations on allowable strains, since fatigue and durability issues may arise if too much thinning during forming occurs.
With the advances in simulation technology to predict the location of potential problems and address them before tool construction begins, the primary emphasis has shifted to accuracy and consistency of product dimensions. These dimensional problems are a function of the elastic stresses created during the forming of the part and the relief of these stresses, or lack thereof, during the unloading of part after each forming operation.
These dimensional problems or springback have always existed in sheet metal forming. However, the magnitude of springback increases as the yield strength of the steel increases. As Advanced High-Strength Steels (AHSS) usage expands due to the combination of higher strength and ductility (for enhanced formability characteristics), countering springback relative to final part dimension becomes critical. First, the design of many of the panels results in higher flow stresses, which are the combination of yield strength and work hardening during deformation. This creates higher elastic stresses in the part. Second, applying AHSS for weight reduction also requires the application of thinner sheet metal that is less capable of maintaining part shape. Third, until recently, most companies had little prior experience applying springback management procedures to their parts made from AHSS. Companies have attacked springback problems with proprietary in-house compensation procedures developed over years of trial and error in the production of various parts. An example would be specific over-crowning of a hood panel or over-bending a simple channel to allow the parts to springback to part print dimensions.
For a given part shape and sheet thickness, the springback occurring when using AHSS grades is greater than that experienced in mild or conventional HSLA steels. The magnitude of springback is a function of the as-formed flow stress, which is the strength of the sheet metal after forming. The as-formed flow stress is a function of the starting yield strength in the flat sheet as well as the work hardening which occurs from forming. Both of these are higher in AHSS grades compared with mild or conventional HSLA steels, and is the basis for the increased springback seen with AHSS.
Figure 1 shows an example of this difference, where two channels of different grades were formed sequentially in a draw die with a pad on the post. The draw die was developed to attain part print dimensions with HSLA 350/450 steel. Strain distributions and lengths of line were nearly identical between the two parts. However, steel property differences between DP and HSLA steels led to different stress distributions, resulting in different dimensional accuracy.

Figure 1: Two channels made sequentially in the same die, with different mechanical properties leading to different springback.
Origins of Springback
The shape of a formed part in its free unconstrained state always deviates somewhat from the shape of punch and die after removal from the tooling. This dimensional deviation between the constrained shape within the tools under full load and after elastic recovery occurs after removing the part from the tool is known as springback.
Stress-strain curves can illustrate how springback changes with material, yield strength, and work hardening. In addition to a tensile test, a stress-strain curve also shows the response of sheet metal as press load is applied to convert it from a flat blank to a formed part.
The blank starts off at the point labeled O in Figure 2, which is the origin with zero stress and zero strain. With a little loading, there is a linear stress-strain response. All deformation here is elastic, meaning that the blank will remain flat after removing the load. All deformation returns to zero providing the applied load stays below the yield strength. Once the yield strength is reached, plastic deformation begins, and the part begins to take shape. Work hardening takes over, with strength increasing as the strain in the part increases. The strength at any given strain is known as the flow stress at that strain.

Figure 2: The magnitude of springback is proportional to the elastic modulus, yield strength and work hardening of the sheet metal, in addition to the part design.E-2
Refer to the left image in Figure 2. Achieving the desired part shape work hardens the part to strength A, occurring at Bottom Dead Center of the press stroke, and resulting in strain C. Removing the part from the constraints of the die under load allows the strains to relax to strain B, relieving the elastic strains. Note how the relaxation follows a path parallel to the initial linear portion of the stress strain curve. The magnitude of BC is a representation of the springback of that part formed to the targeted shape in that location from the selected sheet metal.
Compare that against a steel with higher yield strength and higher work hardening, shown in central image of Figure 2. Achieving the part shape work hardens this steel to strength A’, also reaching strain C since the part shape did not change. After removing this part from the tool, it is free to move to its unconstrained shape. Again, the relaxation follows a path parallel to the initial linear portion. In this case, the strains relax to strain B’. The higher yield strength and work hardening leads to B’C > BC, or a greater amount of springback.
The slope of the initial linear portion of stress-strain curves is known as Young’s Modulus, the Elastic Modulus, or the Modulus of Elasticity. For all steel grades, it is essentially constant at approximately 210 GPa, which is why the slope of that initial section is the same in the left and center images of Figure 2. However, the Elastic Modulus of automotive aluminum alloys is approximately 70 GPa, or ⅓ that of steel. The effect on the stress-strain curve is that the slope of the initial portion decreases by ⅓. This difference in modulus results in aluminum alloys having three times the springback of a similar strength steel, as shown by B’’C in the right image of Figure 2. If higher strength aluminum alloys capable of forming the chosen design exists, springback will be even greater in these parts without making other changes to the product and process.
Although the recovered elastic strains at a given location are relatively small relative to plastic strains in formed parts (on the order of 0.01 % elastic vs. 10 % plastic), they can cause significant shape changes due to its mechanical multiplying effect on other locations when bending deformation and/or curved surfaces are involved. Free edges lack the constraints of the central portion of panels, and therefore are most likely at risk for dimensional issues.
The tooling and component geometry also influence the magnitude of springback. When part geometry prevents complete unloading (relaxing) of the elastic stresses, residual stresses is the term for the constrained elastic stresses remaining in the part. The part takes whatever shape it can to minimize the total remaining residual stresses, either through twisting, bending, or other metal motion. Door panels present an example. The panel coming out of the draw die may have the desired dimensions, but challenges may arise after punching the window cutout and other access holes.
Methods for correcting springback are described here.
Types of Springback
Three modes of springback commonly found in channels and underbody components are angular change, sidewall curl, and twist.
Angular Change
Angular change, or springback, is the angle created when the bending edge line (the part) deviates from the line of the tool. The springback angle is measured as the deviation from the punch radius (Figure 3). If there is no sidewall curl, the angle is constant up the wall of the channel.
Angular change results from the stress difference in the sheet thickness direction when the sheet metal bends over a radius during forming. This stress difference in the sheet thickness direction creates a bending moment at the bending radius. Eliminating or minimizing the angular change requires elimination or minimization of this bending moment.

Figure 3: Schematic showing difference between angular change and sidewall curl.
Sidewall Curl
Sidewall curl is the curvature created in the side wall of a channel (Figures 1 and 3). This curvature occurs when a sheet of metal is drawn over a die/punch radius or through a draw bead. The primary cause of this curl is an uneven stress distribution or stress gradient through the thickness of the sheet metal generated during the bending and unbending process.
During the bending and unbending sequence, the deformation histories for both sides of the sheet are unlikely to be identical due to the differing degrees of tension and compression on each surface. This usually manifests itself by flaring of the flanges, which is an important area for joining to other parts. The resulting sidewall curl can cause assembly difficulties for rail or channel sections that require tight tolerance of mating faces during assembly. In the extreme, a gap resulting from the sidewall curl can be so large that welding is not possible.
Figure 4 illustrates in detail what happens when drawing a sheet metal over the die radius. This bending-unbending process on side A changes from tension (A1) during bending to compression (A2) during unbending. In contrast, the deformation on side B changes from compression (B1) to tension (B2) during bending and unbending. As the sheet enters the sidewall, side A is in compression and side B is in tension, although both sides may have similar amounts of strain. Once the punch is removed from the die cavity (unloading), side A tends to elongate and side B to contract due to the elastic recovery causing a curl in the sidewall.

Figure 4: Origin and mechanism of sidewall curl.
The main source of variation in sidewall curl along the wall comes from the difference in elastic recovery on sides A and B. The higher the strength of the deformed metal, the greater the magnitude and difference in elastic recovery between sides A and B and the associated increase in sidewall curl. The strength of the deformed metal depends not only on the as-received yield strength, but also on the work hardening capacity. This is one of the key differences between conventional HSLA and AHSS. Minimizing the sidewall curl requires minimizing the stress gradient through the sheet thickness.
Strain hardening differences between conventional HSLA and AHSS explain how the relationship between angular change and sidewall curl can alter part behavior. Figure 5 shows the crossover of the true stress – true strain curves when comparing two steels of equal tensile strengths, noting the AHSS grade has a lower yield strength than the conventional HSLA grade.

Figure 5: Schematic description of the effect of hardening properties on springback.K-4
At the lower strain levels usually encountered in angular change at the punch radius, AHSS grades have a lower level of stress and therefore less springback. The predominant trend is increasing angular change for increasing steel strength, as shown in Figure 6, which highlights a nearly linear relationship between tensile strength and angular change.

Figure 6: Angular change increases with tensile strength.K-4
Sidewall curl is a higher strain event because of the bending and unbending of the steel going over the die radius and any draw beads. For the portions of the two stress–strain curves shown in Figure 5, the AHSS grade now is at a higher stress level with increased elastic stresses. Therefore, the sidewall curl is greater for the AHSS grade, as indicated in Figure 7.

Figure 7: Sidewall curl increases with tensile strength.N-2
Remember that AHSS grades have lower yield strength at a given tensile strength. If Figure 5 compared instead a conventional high strength steel grade and an AHSS grade at the same yield strength rather than the same tensile strength, the stress strain curve for the AHSS grade would plot above that for the conventional HSLA grade. In this comparison, the AHSS channel will have greater springback for both angular change and sidewall curl compared to the HSLA channel, and would appear similar to Figure 1.
These phenomena are dependent on many factors, such as part geometry, tooling design, process parameter, lubrication, and material properties. However, higher work-hardening of DP and TRIP steels causes greater increases in the strength of the deformed steel for the same amount of strain.
Any differences in tool build, die and press deflection, location of pressure pins, and other inputs to the stamping can cause varying amounts of springback – even for what should be completely symmetrical parts.
Twist
Twist occurs when two cross sections within the same part rotate differently along their axis, and results from torsion moments in the cross section of the part. The torsional displacement (twist) develops because of unbalanced springback and residual stresses acting in the part to create a force couple, which tends to rotate one end of the part relative to another. As indicated in Figure 8, the torsional moment can come from the in-plane residual stresses in the flange, the sidewall, or both.

Figure 8: Torsion Moment created flange or sidewall residual stresses.Y-2
The actual magnitude of twist in a part is determined by the relationship between unbalanced stresses on the part and the stiffness of the part in the direction of the twist. Low torsional stiffness values in long, thin parts are the reason high aspect ratio parts have significantly higher tendencies to twist. There is also a lever effect, whereby the same amount of twist will result in a larger displacement in a long part than would be the case in a shorter part with a similar twist angle. Twisting is more prone to occur in a thin sheet metal component with large differences in sectional dimensions, such as rails and shallow panels with nearly flat surfaces.
Overcoming the tendency for parts to twist requires reducing the imbalance in the residual stresses forming the force couple that creates the torsional movement. Unbalanced forces are more likely in unsymmetrical parts, parts with wide flanges or high sidewalls, and in parts with sudden changes in cross section. Parts with unequal flange lengths or non-symmetric cut outs are susceptible to twist due to unbalanced springback forces generated by these non-symmetrical features.
Even in geometrically symmetrical parts, unbalanced forces can be generated if the strain gradients in the parts are non-symmetrical. Some common causes of non-symmetrical strains in symmetrical parts are improper blank placement, uneven lubrication, uneven die polishing, uneven blankholder pressure, misaligned presses, or broken/worn draw beads. These problems will result in uneven material draw-in with higher strains and higher elastic recoveries on one side of the part compared to the other, thereby generating a force couple and inducing twist.
Twist can also be controlled by maximizing the torsional stiffness of the part – by adding ribs or other geometrical stiffeners or by redesigning or combining parts to avoid long, thin sections that will have limited torsional stiffness. Minimize twisting potential by:
- Avoiding sudden changes of cross section shape.
- Equalizing the forming depth where possible.
- Optimizing the blank shape to balance deformation.
- Minimizing the flange length normal to the part.
- Incorporating geometric stiffeners like beads or additional flanges.
- Modifying the trim process and sequence to balance stresses.
Global Shape Change
Global shape changes, such as reduced curvature when unloading Class-A surface panel in the die, are usually corrected by the springback management measures described below. The key problem is minimizing springback variation during the run of the part and during die transition. One study showed that the greatest global shape (dimensional) changes initiated from inconsistent die setting practices.A-40
Surface Disturbances
Surface disturbances on Class-A surface panels develop from the reaction to local residual stress patterns within the body of the part. Common examples are high and low spots, oil canning, and other local deformations that form to balance total residual stresses to their lowest value.
Case Study in Springback PredictionO-4
Accurately modeling springback requires knowledge of the yield strength and the hardening behavior during the non-linear strain path followed by each element of the part as the flat blank deforms to the final shape. Part of the challenge stems from the bending-unbending sequence as the sheet metal passes over beads and die radii, leading to what is known as the Bauschinger effect. The Bauschinger effect causes the yield strength to decrease each time the sheet undergoes the tension-compression associated with each bend-unbend, explained graphically in Figure 9.

Figure 9: Graphical explanation of Bauschinger Effect.
In addition to the reduced yield strength from each bend-unbend, the Chord Modulus also decreases each time due to dislocation density evolution, which increases very quickly at the beginning of the plastic deformation. This reduction can be as much as 20% from the value determined in the as-produced steel, as shown in Figure 10.

Figure 10: Chord Modulus decreases after bending-unbending.
The basic mechanism of the Bauschinger Effect is related to the dislocation restructuring during nonlinear deformation, which means it is a function of the steel grade, part shape, and forming process design. These variables make it impossible to have one generic interpretation applicable for all grades and parts. Instead, detailed testing is needed for accurate application of the information.
Modeling these effects accurately is critical in achieving satisfactory springback predictions. A basic isotropic hardening model is insufficient for most stampings, since it assumes that strength is the same in tension and compression – in other words, that there is no Bauschinger Effect. This approach is certainly simpler, but cannot predict springback since it does not reflect physical reality.
Kinematic hardening models, such as the Yoshida‐Uemori (YU) model, do account for the Bauschinger Effect, but this type of model is best applied in linear sections where there is a simple reversal in strain path.Y-7,Y-8
However, many challenging parts have stretch flange sections and curved walls, like seen in the B-Pillar of Figure 11. In this figure, using the YU model for the areas in green leads to inaccuracies due to the multi-directional metal flow.

Figure 11: Using the YU model for the cross loading seen in circled area leads to inaccurate springback predictions.
To account for this cross-loading seen in curved walls, a Homogeneous Anisotropic Hardening (HAH) model was developed.B-10, L-19 Although the specific details of these models are beyond the scope of this article, the yield surface of the YU model consists of three surfaces translated dependently, where instead the yield surface of the HAH model is homogeneous and distorted.
The models were benchmarked against S-Rail test samples made from 1.4mm thick martensitic steel with a tensile strength of 1500 MPa, indicated in Figure 12.

Figure 12: Benchmarked samples made from 1.4 mm martensitic steel with 1500MPa tensile strength.
Material parameters for the YU model and the HAH model were identified and optimized with tension-compression-tension experiments. As shown in Figure 13, the HAH Model provided a better dimensional match to the physical samples.

Figure 13: Homogeneous Anisotropic Hardening (HAH) model matches physical test samples better than the YU model.
Key Points
The potential for springback (angular change, sidewall curl, and twist) increases as the flow stress (the strength after forming) increases. AHSS parts are at greater risk of springback, since parts made from AHSS have high flow stresses arising from their higher strength as produced at the steel mill combined with higher work hardening characteristics compared with conventional HSLA grades.
Sharp bend radii, tight die clearance, and higher binder pressure minimize springback and springback variation. However, formability characteristics of AHSS grades make simultaneously achieving these design features more challenging. Press capability must be sufficient to apply the higher binder pressure, and well as satisfying the necessary forming load and energy requirements.
Drawing or stretching over a radius increases springback. A forming die with an upper pad minimizes drawing over a radius in channel shaped parts, but the risk of sidewall curl exists. When draw forming, ensure sufficient restraining force in the binder with the use of higher blank holding force or draw/lock beads which increase sidewall tension. Stretch-forming produces a stiffer panel with less springback than drawing.
Asymmetric parts and part design promote the conditions for a part to twist by increasing the torsion moment associated with the residual stress in the flange and sidewall. Twisting potential increases with AHSS grades.
Achieving dimensional precision may require multiple stage forming processes or secondary operations. A crown existing in the first step of a channel section may need a second die for flattening and eliminating sidewall springback. Remember that each step work hardens the steel to higher strength levels, reducing the formability and increasing press load and energy requirements.
Methods for correcting springback are described here. The testing needed to calibrate advanced material models for improved springback simulation are described here.
Back To Top









